Our new book (NAT)
Nonabelian algebraic topology: filtered spaces, crossed complexes, cubical homotopy groupoids,
EMS Tracts in Mathematics vol 15
uses mainly cubical, rather than simplicial, sets. The reasons are explained in the Introduction: in strict cubical higher categories we can easily express
algebraic inverse to subdivision,
a simple intuition which I have found difficult to express in simplicial terms. Thus cubes are useful for local-to-global problems. This intuition is crucial for our Higher Homotopy Seifert-van Kampen Theorem, which enables new calculations of some homotopy types, and suggests a new foundation for algebraic topology at the border between homotopy and homology.
A further reason for the connections is that they enabled an equivalence between
crossed modules and certain double groupoids, and later, crossed complexes and strict cubical $\omega$-groupoids.
Also cubes have a nice tensor product and this is crucial in the book for obtaining some homotopy classification results. See Chapter 15.
I have found that with cubes I have been able to conjecture and in the end prove theorems which have enabled new nonabelian calculations in homotopy theory, e.g. of second relative homotopy groups. So I have been happy to use cubes until someone comes up with something better. ($n$-simplicial methods, in conjunction with cubical ideas, turned out, however, to be necessary for proofs in the work with J.-L. Loday.)
See also some beamer presentations available on my preprint page.
Here is a further emphasis on the above point on algebraic structures: consider the following diagram:
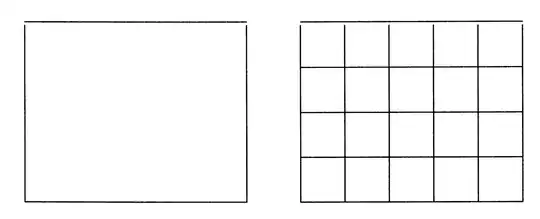
From left to right pictures subdivision; from right to left pictures composition. The composition idea is well formulated in terms of double categories, and that idea is easily generalised to $n$-fold categories, and is expressed well in a cubical context. In that context one can conjecture, and eventually prove, higher dimensional Seifert-van Kampen Theorems, which allow new calculations in algebraic topology. Such multiple compositions are difficult to handle in globular or simplicial terms.
The further advantage of cubes, as mentioned in above answers, is that the formula $$I^m \times I^n \cong I^{m+n}$$ makes cubes very helpful in considering monoidal and monoidal closed structures. Most of the major results of the EMS book required cubical methods for their conjecture and proof. The main results of Chapter 15 of NAT have not been done simplicially. See for example Theorem 15.6.1, on a convenient dense subcategory closed under tensor product.
Sept 5, 2015: The paper by Vezzani arxiv::1405.4548 shows a use of cubical, rather than simplicial, methods, in motivic theory; while the paper by I. Patchkoria, HHA arXiv:1011.4870, Homology Homotopy Appl.
Volume 14, Number 1 (2012), 133-158, gives a "Comparison of Cubical and Simplicial Derived Functors".
In all these cases the use of connections in cubical methods is crucial. There is more discussion on this mathoverflow. For us connections arose in order to define commutative cubes in higher cubical categories: compare this paper.
See also this 2014 presentation The intuition for cubical methods in algebraic topology.
April 13, 2016. I should add some further information from Alberto Vezzani:
The cubical theory was better suited than the simplicial theory when dealing with (motives of) perfectoid spaces in characteristic 0. For example: degeneracy maps of the simplicial complex $\Delta$ in algebraic geometry are defined by sending one coordinate $x_i$ to the sum of two coordinates $y_j+y_{j+1}$. When one considers the perfectoid algebras obtained by taking all $p$-th roots of the coordinates, such maps are no longer defined, as $y_j+y_{j+1}$ doesn't have $p$-th roots in general. The cubical complex, on the contrary, is easily generalized to the perfectoid world.
November 29, 2016 There is more information in this paper on Modelling and Computing Homotopy Types: I which can serve as an introduction to the NAT book.
February 26, 2020 Theorem 5.4.7 of the NAT book is a generalisation of a result of JHC Whitehead on free crossed modules, in the form of a complete description of the crossed module $\pi_2(X \cup_f CA,X,x) \to \pi_1(X,x)$ in terms of the morphism $f_*:\pi_1(A,a) \to \pi_1(X, x)$. The proof in the NAT book uses cubical methods. (Whitehead's case was $A$ is a wedge of circles.) Thus we need to look at comparisons of a reasonably sophisticated level of applications.
