The recently discovered amazing aperiodic monotile (or "einstein") of David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss tiles the plane only if reflections of the monotile are allowed. Is there hope of modifying their construction to produce an aperiodic monotile that tiles the plane without the need for reflected tiles?
Asked
Active
Viewed 1,325 times
22
-
1Would a shape count if there were periodic tilings of $\mathbb{R}^2$ by it, but every such tiling used tiles related by a reflection? – Rosie F Mar 28 '23 at 10:39
-
@RosieF Interesting question. What's an example of a monotile (which may or may not tile the plane non-periodically) with such a property? – Timothy Chow Mar 28 '23 at 22:36
-
3Doris Schattschneider shows families of pentagons which tile, and marks tiles that are "flipped". Michael Rao is an updated, more technical paper. Joseph Myers has stats on suitable polyforms. Each link in the "isohedral" & "anisohedral" columns links to a file of shapes which tile but not by only translation & 180 degree rotation. Some tile but only with reflection. – Rosie F Mar 29 '23 at 06:35
1 Answers
20
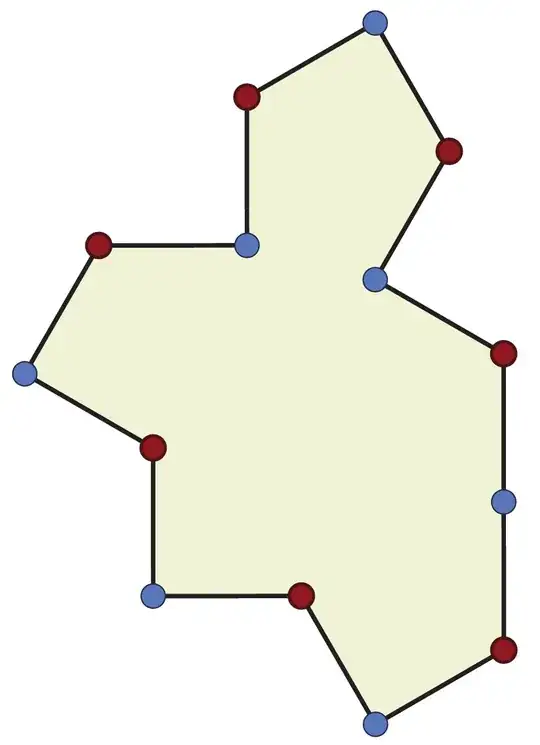
The same authors have just released a preprint claiming a positive answer to this question.
EDIT: Here is a picture of the reflection-free aperiodic monotile:
More visualizations and other data are available at this web page of one of the authors.
Terry Tao
- 108,865
- 31
- 432
- 517
-
2NIce. If I understand correctly, this paper provides examples of the type suggested in Rosie F's comment above as well as examples satisfying a stricter aperiodicity criterion. – Timothy Chow May 30 '23 at 03:50