Number of real roots of 0,1 polynomial

Question

$0,1$ polynomial has coefficients from $\{0,1\}$. I investigate the number of roots in such polynomials.
We are talking about real roots, and multiples are counted only once.
It was found numerically that minimal degrees $\nu(n)$ of such polynomials with given number of roots $n$ are (07.03):
(In the light of @Timothy Chow answer $\sqrt{\nu(n)}/n$ column added)

$n$	$\nu(n)$	$\sqrt{\nu(n)}/n$	Example
1	1	1.0	$x$
2	2	0.707	$x^2+x$
3	7	0.882	$x^7+x^4+x^2+x$
4	10	0.791	$x^{10}+x^9+x^7+x^4+x^2+x$
5	19	0.872	$x^{19}+x^{18}+x^{16}+x^{11}+x^9+x^6+x^5+x^4+x^2+x$
6	28	0.882	$x^{28} + x^{27} + x^{25} + x^{20} + x^{18} + x^{14} + x^{13} + x^{11} + x^{9} + x^{8} + x^{7} + x^{4} + x^{2} + x$
7	$\le$41	$\le$0.915	$x^{41} + x^{40} + x^{38} + x^{35} + x^{31} + x^{30} + x^{29} + x^{24} + x^{20} + x^{18} + x^{17} + x^{15} + x^{12} + x^{11} + x^7 + x^4 + x^2 + x$
(7)	$\le$45	$\le$0.958	$x^{45} + x^{44} + x^{42} + x^{37} + x^{35} + x^{33} + x^{31} + x^{30} + x^{28} + x^{26} + x^{24} + x^{23} + x^{22} + x^{20} + x^{18} + x^{16} + x^{15} + x^{13} + x^{11} + x^{9} + x^{4} + x^{2} + x$
8	$\le$52	$\le$0.944	$x^{52} + x^{51} + x^{49} + x^{46} + x^{40} + x^{38} + x^{37} + x^{36} + x^{35} + x^{33} + x^{31} + x^{29} + x^{27} + x^{26} + x^{24} + x^{22} + x^{20} + x^{18} + x^{17} + x^{16} + x^{15} + x^{13} + x^{7} + x^{4} + x^{2} + x$

And are there some theoretical results about real roots of $0,1$ polynomials?

Updates

1

OEIS' editors decided to separate these results into a new sequence:
A368824
Anyone can edit and update it!

2

All polynomials with the maximum number of roots except $p_1$ (which is understandable) and $p_7$ (which I think will be corrected) have roots $0$ and $-1$ and their plots on $[-1,0]$ look like this:

I've got mad idea that there is some differential equation
$P(x)y''(x) + Q(x)y'(x) + R(x)y(x)=0$
with boundary conditions $y(0)=y(-1)=0$ which is related to these polynomials...

3

A solution was added for $n=7$ with degree $\nu=41$, see Fred's answer below. This polynomial has a root at $x=-1$ as expected. I did not yet delete the prevoius solution with $\nu=45$.

Answer 1

And are there some theoretical results about real roots of 0,1 polynomials?

Such polynomials are called Newman polynomials. One commonly cited reference is Zeros of polynomials with 0,1 coefficients by A. Odlyzko and B. Poonen, L’Enseignement Mathématique 39 (1993), 317–348. In the paper Littlewood-type problems on [0,1] by P. Borwein, T. Erdélyi, and G. Kós, Proc. London Math. Soc. 79 (1999), 22–46 (DOI), the authors show:

There is an absolute constant $c > 0$ such that every polynomial $p$ of the form $$p(x) = \sum_{j=0}^n a_j x^j, \quad |a_j| \le 1, \quad |a_0| = |a_n| = 1, \quad a_j \in \mathbb{C}$$ has at most $c\sqrt{n}$ real zeros.

This result gives some information about the question you asked, though it does not answer it completely. There is a large literature on Newman polynomials and their close cousins, the Littlewood polynomials (whose coefficients are $0$ or $\pm1$); see for example K. G. Hare and J. Jankauskas, On Newman and Littlewood polynomials with prescribed number of zeros inside the unit disk (arXiv:1910.13994) or T. Erdélyi, On the zeros of polynomials with Littlewood-type coefficient constraints (Michigan Math. J. 49 (2001), 97–111) and the references therein.

Answer 2

This is not an answer, just a response to David's request to present a construction of a polynomial $P(x)=\sum_{j=0}^d a_jx^j$ of arbitrarily high degree $d$ whose coefficients are real, bounded by $1$ in absolute value, satisfy $|a_0|=|a_d|=1$, and whose number of distinct real roots is of order $\sqrt{d}$. I believe that I've also seen the statement about pure $0,\pm 1$ coefficients somewhere, but my attempt to retrieve the proof from my memory or to reinvent it failed, so it might be a hallucination.

We will use the standard "pigeonholing with a minor correction in the end" mumbo-jumbo.

Take big $n>m,k$

Let $P(x)=exT(x)$ where $T$ is the Chebyshev's polinomial of degree $m$ adjusted to the interval $[e^{-1},1]$. Then $P$ has no free term, is of degree $m+1$, and has the alternance points $y_1,\dots,y_{m+1}$ of heights at least $1$. The coefficients of $P$ are bounded by $K^m$ for some absolute $K>0$. Let $x_j=y_j^{1/k}$

Consider all polynomials $Q$ of degree $3n$ with coefficients $\beta_s\in{0,e^{(s/(3n))^2}}$. Since $|Q(x_j)|\le 3en$ for every $Q$ and every $j$, and the total number of $Q$-s is $2^{3n}$ we can (by pigeonholing) find a set of $2^{2n}$ $Q$-s such that their values at each $x_j$ are confined to some intervals $I_j$ of length $6en2^{-n/m}$.

Fix one such $Q_0$ in that set. Note that the number of $Q$ whose coefficients differ from those of $Q$ only within an interval of indices of length $n$ is at most $n2^n\ll 2^{2n}-1$. Thus we can find $Q_1$ in the same set such that $$ R(x)=Q(x)-Q_1(x)=\sum_{s=a}^b \alpha_s x^s $$ with $b>a+n$, $\alpha_s\in\{0,\pm e^{(s/(3n))^2}\}$, $\alpha_a,\alpha_b\ne 0$, $|R(x_j)|\le 6en2^{-n/m}$ for all $j$.

Dividing $R(x)$ by $e^{(a/(3n))^2}x^a$, and taking into account that $x_j\ge e^{-1/k}$ for all $j$, we get a polynomial $S(x)$ of degree $d=b-a\in[n,3n]$ with coefficients $\gamma_s\in\{0,\pm e^{\psi(s)}\}$ where $\psi(s)=[(s+a)/(3n)]^2-[a/(3n)]^2$ and such that $|S(x_j)|\le 6en 2^{-n/m}e^{3n/k}$.

The key point is that $\psi$ is strictly convex and $0$ at $0$, so $$ e^{\psi(s)}\le e^{s\psi(d)/d}-\kappa n^{-2} $$ for $1\le s\le d-1$ with some $\kappa>0$. This we can correct the intermediate coefficients by about $n^{-2}$ and still have them under the geometric progression joining $|\gamma_0|=1$ and $|\gamma_d|=e^{\psi(d)}$. Then, by a trivial linear change of variable, we'll turn $\gamma_d$ into $\pm 1$ and make all intermediate coefficients $\le 1$.

We will use this correction possibility to turn smallness at $x_j$ into an alternance. To this end, we will just add $ 6en 2^{-n/m}e^{3n/k}P(x^k)$. The coefficients of this correction are bounded by $6en 2^{-n/m}e^{3n/k}K^m$, it has no free term and the degree $(m+1)k$.

Now choose $k\approx \sqrt n$ and $m\approx \sigma\sqrt n$. Then if $\sigma<1$, our correction stays inside the degree span of $S$, and $$ 6en 2^{-n/m}e^{3n/k}K^m\approx 6en 2^{-\sigma^{-1}\sqrt n}e^{3\sqrt n}K^{\sigma\sqrt n}\, $$ which for small $\sigma>0$ is less than $e^{-\sqrt n}\ll \kappa n^{-2}$, so our correction has admissible size too. If you want both endpoint coefficients to be $1$ and they are $1$ and $-1$ in the polynomial we constructed, just multiply by $1-x^{d+1}$ doubling the degree.

That's it. You can see, however, that the alternance is minuscule, so you should exercise utmost care with your suggested replacement of negative coefficients, if it can work at all!

Edit: For completeness, let me also give a short proof that a polynomial $P(x)=\sum_{j=0}^na_j x^j$ with real coefficients $a_j$ satisfying $|a_j|\le 1$, $|a_0|=|a_n|=1$ cannot have more than $C\sqrt n$ real roots.

By considering $P(\pm x)$, $x^nP(\pm 1/x)$, we see that it suffices to bound the number of roots on $(0,1]$.

Recall that the Chebyshev polynomial $T_d(y)=\frac 12[(x-\sqrt{x^2-1})^d+(x+\sqrt{x^2-1})^d]$ with even $d$ has all its roots real, is bounded by $1$ on $[-1,1]$, is decreasing on $(-\infty,1]$ and is greater than $4$ at $-1-\frac C{d^2}$ for some absolute constant $C>0$. After proper rescaling and taking $Cd^{-2}\approx\delta\in(0,\frac 12)$, it turns into a polynomial $S_\delta(z)$ on $[0,1]$ such that the degree of $S$ is $\le C\delta^{-1/2}$, $|S(z)|\le S(0)=1$ for every $z\in[0,1]$ and $|S(z)|\le \frac 14$ when $z\in[\delta,1]$.

Now choose $\delta=\frac 1n$ and put $$ Q(z)=S_\delta(z/n)S_{2\delta}(z/n)S_{4\delta}(z/n)\dots S_{2^m\delta}(z/n) $$ where $2^m\delta\in[\frac 14,\frac 12)$. Then $Q$ still has all roots real, the degree of $Q$ is at most $C\sqrt n\sum_{j\ge 0}2^{-j/2}$, $Q(0)=1$ and $Q(k)\le 4^{-q-1}$ for $k\ge 2^q$ for $0\le q\le m$. The last estimate implies that $\sum_{k=1}^n |Q(k)|<1=Q(0)$ for not too small $n$. Hence, in the polynomial $$ \widetilde P(x)=\sum_{k=0}^n a_kQ(k)x^k $$ the constant term dominates the sum of all other ones, so $\widetilde P$ has no roots on $(0,1]$.

Now write the factorization $Q(z)=a\prod_s(z-\lambda_s)$, $\lambda_s\in\mathbb R$. Then $$ \widetilde P=a\left[\prod_s D_{\lambda s}\right]P $$ where $D_\lambda f=xf'-\lambda f$ is the differential operator that acts on the polynomials by multiplying the $k$-th coefficient by $k-\lambda$.

Representing $D_\lambda f=x^{\lambda+1}\frac{d}{dx}[x^{-\lambda}f]$ and using Rolle's theorem, we conclude that each application of $D_\lambda$ can reduce the number of roots of $f$ on $(0,1]$ by at most $1$. But the degree of $Q$ is only $C\sqrt n$ and we have lost all roots of $P$ on $(0,1]$ when passing from it to $\widetilde P$. The end.

Answer 3

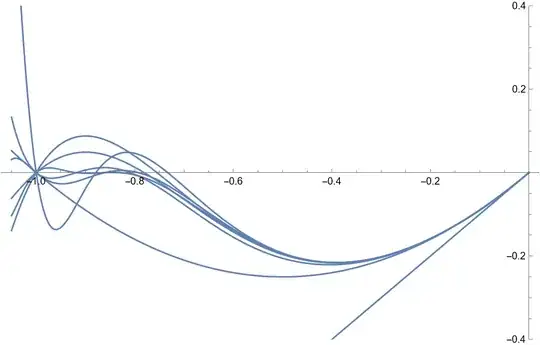
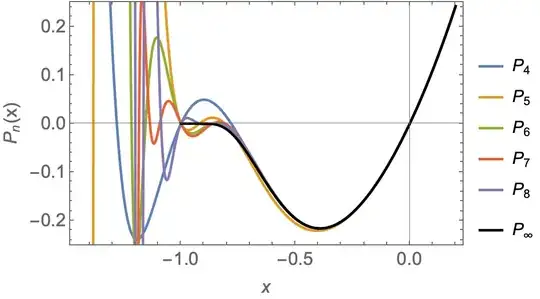
Still, it seems worth recording what you know. I think at this point the question should be treated as "can we figure out anything?" – David E Speyer

Well, OK. Trying to figure out anything is exactly what we are now doing with Zachary Chase, and below is a small observation.

Let $\alpha>0$. Suppose that $n$ is even and we want to construct a polynomial $P_a(x)=\sum_{j=0}^n a_jx^j$ with $a_0=a_n=\alpha$, $a_j\in[0,1]$ for $1\le j\le n-1$ having roots $-1,-x,-x^2,\dots,-x^m$ where $x\in(0,1)$ is some number close to $1$.

It is possible if and only if the convex set $$ E=\{(a_0,a_n,P_a(x^k), 0\le k\le m):\\ a_0,a_n\in \mathbb R, a_j\in[0,1], 1\le j\le n-1\}\subset \mathbb R^{m+3} $$ contains the point $z=(\alpha,\alpha,0,\dots,0)$. So suppose that it does not. Then there is a non-trivial linear functional $\psi$ on $\mathbb R^{m+1}$ that is non-negativeon $E-z$, i.e., we can find some coefficients, not all $0$ such that $$ u(a_0-\alpha)+v(a_n-\alpha)+\sum_{k=0}^m w_kP_a(x_k)\ge 0 $$ for every admissible choice of the coefficient vector $a$.

Introduce the polynomial $Q(z)=\sum_{k=0}^m w_k z^k$. Then the condition above can be rewritten as $$ -u\alpha-v\alpha+(u+Q(1))a_0+(v+Q(x^n))a_n+\sum_{j=1}^{n-1}(-1)^jQ(x^j)a_j\ge 0\,. $$ Now, since $a_0$ and $a_n$ are free to run over the entire real line, we must have $u+Q(1)=v+Q(x^n)=0$. As to the rest of the expression, its minimum equals $$ U=\sum_{j=1}^{n-1} \min[(-1)^jQ(x^j),0]\,. $$ Thus we must have $$ \alpha(Q(1)+Q(x^n))+U\ge 0 $$ for some not identically $0$ polynomial $Q$ of degree at most $m$.

Now, when $x$ is really close to $1$, the points $x_j$, $j=1,\dots,n-1$ make an almost equispaced net on the interval $I=[x^n,1]$. Let $\mu=|Q(z)|=\max_I|Q|$. Then, by Markov's inequality, $|Q'|\le\frac 2{|I|}m^2\mu$ on $I$, so there is an interval of length $\frac{|I|}{4m^2}$ containing $z$ on which $Q$ preserves sign and is at least $\mu/2$ in absolute value. Since the powers $x_j$ are separated by about $|I|/n$, this interval contains about $cn/m^2$ powers $x^j$ with odd and even $j$. Choosing the parity appropriately, we get $U\le -c\frac{n}{m^2}\mu$.

On the other hand, $Q(1)+Q(x^n)\le 2\mu$. Hence we run into a contradiction when $2\alpha m^2\le cn$.

The conclusion: For every fixed $\alpha\ge 1$ and even $n$, there exists a polynomial $P_a(x)$ with $a_0=a_n=\alpha$, $a_j\in[0,1]$ ($j=1,\dots,n-1$) having $m$ distinct roots on $[-1,0)$, provided that $m^2\le c\alpha^{-1} n$.

It means that the non-negativity of the coefficients doesn't impose any substantial additional restrictions on the number of roots compared to the boundedness alone and the whole issue is the discretization from $[0,1]$ to $\{0,1\}$.

Edit I'll continue dumping obvious observations here. We'll now construct a polynomial with coefficients $0$ and $1$ having about $\frac{\log^2 n}{\log\log n}$ roots. While by itself this lower bound is rather pathetic, it still means that one should, probably, concentrate for a while on driving the lower bound up rather than the upper bound down.

The key observation is that $\sqrt[3]2<\frac 43$ by Bernoulli, so $$ -1+\sqrt[3]{\frac 12}+\frac 12-\left(\frac 12\right)^3-\left(\frac 12\right)^9 \\ >-1+\frac 34+\frac 12-\frac 18-\frac 18=0\,. $$ The immediate conclusion is that if $a=(-1,-1,1,1,-1,-1,1,1,\dots)$ and $p_j$ is an increasing sequence of positive integers such that $p_{j+1}\ge 3p_j$ for all $j$, then the polynomial $$ 1+\sum_{k=1}^u a_kx^{p_k} $$ has at least about $u/2$ sign changes on $(0,1)$. To turn it into $0,1$ polynomial with roots on $(-1,0)$, we need also to ensure that the parity of $p_j$ agrees with the sign of $a_j$, of course.

Now fix the (large) target degree $n$. Let $q,u,v$ be positive integers. Let $I_k=[3\cdot 6^{k-1} q, 6^k q]$ ($k=1,\dots,u$).

We will now choose $uv$ pairwise distinct integers $p_{ij}$ ($i=1,\dots,v, j=1,\dots,u$) so that for fixed $i$ one has $p_{ij}\in I_j$ for all $j$ and $p_{ij}$ has appropriate parity to be used in the above construction. We will also require that every positive integer $p$ can be written as the sum of at most $v$ integers $p_{ij}$ in at most one way. To ensure that such choice is possible by the mindless "just-take-what-is-still-available" algorithm, it is enough to require that $(uv)^{2v-1}<q$.

Now just put $P_i(x)=1+\sum_{j=1}^u x^{p_{ij}}$ and $P(x)=\prod_{i=1}^v P_i(x)$. Then $P$ is a $0,1$ polynomial of degree $\le 6^u qv$ and about $uv/2$ roots. To keep the degree under $n$ and to satisfy the previous condition, we choose $q\approx\sqrt n$, $u\approx c\log n$, $v\approx c\frac{\log n}{\log\log n}$ with sufficiently small $c>0$.

To be completely honest, I should also ensure that the roots of different $P_i$ are different too, but with that much freedom in choosing $p_{ij}$ that is rather trivial, so I'll leave it as an exercise to the interested readers.

Edit 2: Some more "Mathematische Banalen". This time we will show the existence of a polynomial with coefficients $0,\pm 1$ having $c\frac{\sqrt n}{\log n}$ zeroes on $[0,1]$. This still falls a bit short of $\sqrt n$, but is way better than $n^{1/4}$ David found in the literature for this case.

We start with a few observations about such polynomials. First, if $P$ is such a polynomial of degree $n$, then $$ \int_0^1 |P(x)|\,dx\ge n^{-C\sqrt n} $$ for large $n$ unless $P$ is identically $0$.

Indeed, if we use the same polynomial $Q$ and the differential operators $D_\lambda$, we will see that applying $D_{\lambda_s}$ with various $\lambda_s\in[0,n]$ to $P$ at most $C\sqrt n$ times, we'll get a polynomial $\widetilde P$ in which the first non-zero coefficient is $\pm 1$ and the sum of the absolute value of all other coefficients is below $1/2$. Then $\int_0^1|\widetilde P(x)|\,dx\ge\frac 12\int_0^1 x^n\,dx=\frac 1{2(n+1)}$.

However, due to the Markov's inequality $\|P'\|_\infty\le 2n^2\|P\|_\infty$, we have that the $L^1$ and the $L^\infty$ norms of $P$ are equivalent up to a factor $Cn^2$. Moreover, $|P|\ge \frac 12\|P\|_\infty$ on an interval of length $cn^{-2}$ around the point of the maximum of $P$. Thus, we have $\|P'\|_1\le Cn^4\|P\|_1$ and $\|P\|_\infty\le Cn^2 \int_{[0,1]\setminus E}|P|$ for every set $E$ of meaure $|E|\le cn^{-2}$.

The first conclusion shows that every application of $D_\lambda$ increases the $L^1$ norm of a polynomial of degree $n$ at most $Cn^4$ times, which immediately implies our first observation.

Now consider all polynomials $R$ of degree $n$ with coefficients $0,1$ and for each of them kompute their first $m+1$ moments $\int_0^1 R(x)x^k\,dx$ ($k=0,\dots,m$). Those are numbers in $[-n-1,n+1]$. Using the pigeonhole principle, as usual, and shamelessly exploiting the fact that the difference set is exactly what we need, we find a polynomial $P$ with coefficients $0,\pm 1$ that is not identically $0$ but satisfies $$ \left|\int_0^1 P(x)x^k\,dx\right|\le 2(n+1)2^{-\frac nm}\,. $$ for all $k=0,\dots,m$.

Now assume that $P$ has only $u<m$ distinct roots $r_j$ on $[0,1]$ at which a crossing occurs. Consider $q(x)=\prod_j(x-r_j)$. That is a polynomial of degree $u<m$ with the sum of absolute values of its coefficients at most $2^m$. Thus $$ \left|\int_0^1 P(x)q(x)\,dx\right|\le 2(n+1)2^{-\frac nm}2^m\,. $$

On the other hand, $Pq$ preserves sign and $|q|\ge (c'n^{-2})^m$ outside a set $E\subset \mathbb R$ of measure $|E|<cn^{-2}$ (Cartan's lemma). Hence the LHS equals $$ \int_0^1|P||q|\ge\int_{[0,1]\setminus E}|P||q|\ge (c'n^{-2})^m cn^{-2}\|P\|_\infty\ge (c'n^{-2})^m cn^{-2} n^{-C\sqrt n}\,, $$ so if $$ (c'n^{-2})^m cn^{-2} n^{-C\sqrt n}>2(n+1)2^{-\frac nm}2^m\,, $$ which happens for $m=c\frac{\sqrt n}{\log n}$, we get a contradiction.

Next Edit:

Let's now construct (or, rather, prove the existence of) a non-zero polynomial $P$ with coefficients $0,\pm 1$ of degree at most $n$ that has at least $c\sqrt n$ roots on $(0,1)$. This construction will, finally, justify my casual remark in the beginning of this long discussion (which I still hope to continue) and show that my memory, albeit failing, can still be occasionally trusted somewhat. If you ask me when, where, and by whom this argument was first invented, I have no idea.

We'll proceed as before but do everything way more carefully. First, we shall show that if $a>0$ is a small number, then every polynomial $P(z)=\sum_{k=0}^n a_kz^k$ with coefficients $a_k\in [-1,1]$ and $a_0=\pm 1$ satisfies $\|P\|_{L^0(I)}\ge \exp(-C/a)$ where $I=[1-2a,1-a]$ and for a non-negative function $f$ on an interval $J$, $\|f\|_{L^0(J)}=\exp\left[\frac{1}{|J|}\int_J\log| f|\right]$ is the geometric mean of $f$ on $J$.

This is achieved by considering the domain $\Omega$ that is a disk centered at some point $z_0\in(0,\frac 13)$ (say, $z_0=\frac 16$) of radius $1-|z_0|$ with a slit $[1-3a,1]$ (the blue circle on the figure below).

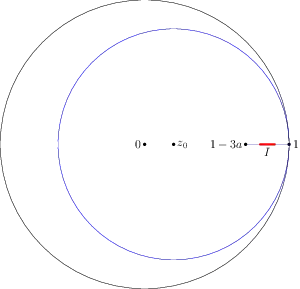
Applying Jensen's inequality, we see that $$ 0 =\log|P(0)|\le\int_{\partial\Omega}\log|P|d\omega $$ where $\omega$ is the harmonic measure on $\partial\Omega$ with respect to $0$. Now we first estimate the integral of $\log_+|P|$ using the trivial bound $|P(z)|\le\frac 1{1-|z|}$. We split $\partial\Omega$ into the slit part $S$ and the circle part $C$. Note that the conformal mapping of $\Omega$ to the unit circle is given by an explicit formula, so the density of $\omega$ can be found exactly, but I still prefer a back of the envelope computation that isn't algebraicly heavy even if it is a bit lengthier.

First, we consider the harmonic function $u(z)=\log\frac 1{|1-z|}$ in $\Omega$. we have $$ 0=u(0)=\int_S\log\frac 1{|1-z|}\,d\omega(z)+\int_C\log\frac 1{|1-z|}\,d\omega(z)\,. $$ Since $\log\frac 1{|1-z|}\ge -\log 2$ on $C$, we conclude that $$ \int_S\log_+|P|\,d\omega\le \int_S\log\frac 1{1-|z|}\,d\omega(z) \\ =\int_S\log\frac 1{|1-z|}\,d\omega(z)\le\log 2\,. $$ Now note that on $C$, the harmonic measure $\omega$ is dominated by the harmonic measure for the disk without a slit, which has bounded density with respect to the Lebesgue measure on the circumference, so the integral of $\log\frac{1}{1-|z|}$ with respect to $\omega$ is uniformly bounded by some constant depending on (fixed) $z_0$ but not on the size of the slit. Thus, $\int_{\partial\Omega}\log_+|P|\,d\omega\le C$ independently of $a$ and, therefore, $\int_I \log_-|P|\,d\omega\le \int_{\partial\Omega}\log_-|P|\,d\omega\le C$ as well.

Now we need a more clear idea of what $\omega$ is on $I$. First, map the disk conformally to the upper half-plane so that $0$ is mapped to $i$ and $1$ to $0$, say. Then the slit $S$ will be mapped to $[0,hi]$ for $h$ comparable to $3a$ and the mapping will be bi-Lipshitz on the slit and $\omega$ will be mapped to the harmonic measure $\omega'$ in the half-plane with the new slit.

Now, we use the usual $w\mapsto\sqrt{w^2+h^2}$. Then we'll get $d\omega'(w)\approx \frac{|w|}{\sqrt{|w^2+h^2|}}|dw|$. When $z$ is in $I$, the corresponding point $w$ is in the "middle part" of $[0,hi]$, so the factor in front of $|dw|$ is comparable to $1$. Thus, on $I$, we have $d\omega(z)\approx |dz|$ and we conclude that $\int_{I}\log_-|P(x)|\,dx\ge -C$, i.e., $\|P\|_{L^0(I)}\ge \exp[-C/|I|]=\exp[-C/a]$.

If $a_0=0$ and $a_k\in\{0,\pm 1\}$, consider the least $m\in[0,n]$ for which $a_m=\pm 1$. The factor $x^m\ge(1-2a)^n\ge \exp[-3an]$ on $I$, so in this case $$ \|P\|_{L^0(I)}\ge\exp\left[-\tfrac Ca-3an\right]\,. $$ We shall now fix $a=\frac 1{\sqrt n}$, so $$ \|P\|_{L^0(I)}\ge\exp\left[-C\sqrt n\right]\,. $$ This part has been definitely known to Borwein and Erdelyi. Now we play the same game as before but only on the interval $I$. Formally, for a polynomial $P$, we define $$ \widetilde P(t)=P(1-a-at), \qquad t\in[0,1]\,. $$ The above result states now that for every non-zero $P$ with coefficients $0,\pm 1$, we have $$ \|\widetilde P\|_{L^0([0,1])}\ge \exp[-C\sqrt n]\,. $$ Now, noting that $\|\widetilde p\|_{L^\infty([0,1])}\le n$ for any $p$ with coefficients $0,1$ and using the pigeonhole as before, we can find a non-zero polynomial $P$ with coefficients $0,\pm 1$ such that $$ \left|\int_0^1 \widetilde P(t) t^\ell\,dt\right|\le n2^{-n/m},\qquad \ell=0,1,\dots,m-1\,. $$ Assuming that $\widetilde P$ has only $m-1$ (or fewer) sign changes on $[0,1]$ (i.e., that $P$ has fewer than $m$ zeroes on $I$), we again construct $Q(t)=\prod_j(t-t_j)$ as before and note that $\widetilde PQ$ preserves sign on $[0,1]$. Also, $\|Q\|_{L^0([0,1])}\ge\exp[-Cm]$ (each factor $|t-t_j|$ has geometric mean uniformly bounded from below. So, we get the chain of inequalities $$ \exp[-C\sqrt n-Cm]\le \|\widetilde P Q\|_{L^0([0,1])}\le\|\widetilde P Q\|_{L^1([0,1])} \\ =\left|\int_0^1 \widetilde P Q\right|\le 2^m n2^{-n/m}\,, $$ and when $m=c\sqrt n$ with small enough $c>0$, we get a contradiction.

Next Edit

The construction of a polynomial with coefficients $0,\pm 1$ having $m$ roots on $(-1,0)$ of degree $Cm^2$ allows one also to construct a polynomial with coefficients $0,1$ of degree $n$ with $c\log^2 n$ roots on $(-1,0)$ giving a slight improvement from the previous "trivial bound" $c\log^2 n/\log\log n$.

To carry the construction out, note first of all that when looking for the sign changes of $P$ in the construction for the $0,\pm 1$ case, we can ignore the humps of height $\exp(-C_1 m)$ with large constant $C_1$ because they can be responsible only for a tiny portion of the $L^1-norm$ of $\widetilde PQ$ ($e^{-C_1 m}$ is much less than $e^{-Cm-C\sqrt{Cm^2}}$). Thus, a polynomial $P$ has not only $m$ roots, but also an alternance of size $m$ and of height $e^{-C_1 m}$.

Now take $P_1(x)=P(x^N)$. Then this alternance occurs on the interval $J=[-1,-1+\frac 1N]$ (actually even a bit shorter one, but it is irrelevant). Consider the polynomial $T(x)=\prod_{k:3^k\le \sqrt N}(1+x^{3^k})-1$. Note that $T$ has coefficients $0,1$ and $|T(x)+1|\le N^{-c\log N}=e^{-c\log^2 N}$ on $J$. Thus, if this number is below $e^{-C_1 m}/(Cm^2)$, we can replace every negative power $-x^k$ in $P_1$ by $x^kT(x)$ without disturbing the alternance too much. That happens exactly when $m<c_1\log^2 N$. The rest should be obvious.

Note that this construction is based exactly on David's idea to take whatever we have and to replace negative coefficients by something else afterwards. This particular realization of the idea raises the degree way too much, however. Any suggestions how to do it better? Remember that we cannot raise the multiplicity of zero at $-1$ substantially (see our discussion with Peter Mueller), so some other approach is needed.

Answer 4

Update 1: I've now used the multiprocessing library for the parallelization of the computation, and accordingly updated the Python code.

---

Update 2: We have $\nu(7)\le 45$ and $\nu(8)\le 52$, as the polynomials \begin{equation} x^{45} + x^{44} + x^{42} + x^{37} + x^{35} + x^{33} + x^{31} + x^{30} + x^{28} + x^{26} + x^{24} + x^{23} + x^{22} + x^{20} + x^{18} + x^{16} + x^{15} + x^{13} + x^{11} + x^{9} + x^{4} + x^{2} + x \end{equation} and \begin{equation} x^{52} + x^{51} + x^{49} + x^{46} + x^{40} + x^{38} + x^{37} + x^{36} + x^{35} + x^{33} + x^{31} + x^{29} + x^{27} + x^{26} + x^{24} + x^{22} + x^{20} + x^{18} + x^{17} + x^{16} + x^{15} + x^{13} + x^{7} + x^{4} + x^{2} + x \end{equation} have $7$ and $8$ real roots, respectively.

These polynomials are the lexicographically smallest ones of the form $xh(x)$, where $h$ is equal to its reciprocal.

---

Below is the Python code which for each sufficiently small integers $n$ computes the maximum number of distinct real roots of degree $n$ polynomials with coefficients $0$ and $1$ (which is [OEIS A362344][1]) together with a lexicographically smallest example. For instance, we see within a few minutes that $\nu(6)=28$. (Initially, I had written a SageMath script using Sturm's Theorem, however the `polsturm` function from pari/gp is somewhat faster.)

The Python script makes use of parallelization. The line cpus = 17 tells that $17$ cores will be used and of course can be adjusted.

The script identifies the binary expansion $p=\sum a_i2^i$ with the polynomial $\sum a_ix^i$.

In case that someone wants to look for a pattern, here is a list of all $0$-$1$-polynomials of degree $28$ with $6$ distinct real roots. To save space, we give the list as the numbers $p$ (see above): 437545878, 437594646, 437594838, 437619606, 437643798, 440494998, 440875158, 443812758, 443836950, 443837334, 443910678, 443911062, 443916438, 443923062, 443923350, 444033942, 450177558, 462908310. (In SageMath, if K is a polynomial ring, and p is one of these numbers, K(p.bits()) yields the corresponding polynomial.)

And here is the Python code (which requires the library cypari2):

import cypari2
from multiprocessing import Pool
pari = cypari2.Pari()
cpus = 17 ################
def check(i):
    best, bestp, bestf = 0, 0, ''
    for p in range(2n + i, 2(n+1), cpus):
        l = []
        for j, b in enumerate(bin(p)[-1:1:-1]):
            if b == '1':
                l.append(f'x^{j}')
        s = '+'.join(l)
        m = pari(s).polsturm()
        if m > best:
            best, bestp, bestf = m, p, s
    return best, bestp, bestf
n = 1
while True:
    n += 1
    with Pool(cpus) as P:
        res = P.map(check, range(cpus))
    best, bestp = 0, 2**(n+1)
    for m, p, s in res:
        if m > best or (m == best and p < bestp):
            best, bestp, bestf = m, p, s
    print(f'deg = {n}, m = {best}, p = {bestp}, bestf = {bestf}')

Answer 5

At last, here is a proper answer. The maximal possible number of real zeroes of a polynomial of degree $n$ with coefficients $0$ and $1$ is comparable to $\sqrt n$ for large $n$.

The overview of the proof

We have already seen in one of the non-answers that $C\sqrt n$ is an upper bound even for a much wider class of polynomials. Now our task is just to outline the construction of a polynomial with about $m$ zeroes of degree about $m^2$ for each large $m$. It will be more convenient for us to make the change of variable $x\mapsto -x$ and to consider the polynomials $$ P_n(x)=1+\sum_{k=1}^n (-1)^k\varepsilon_k x^k,\quad \varepsilon_k\in\{0,1\} $$ with even $n\ge 0$ on the interval $(0,1)$.

Fix $m$. The way we'll force $P$ to have about $m$ zeroes is via the following

Lemma. Let $f$ be a real valued continuous function on an interval $I$. Split $I$ into $m-1$ equal subintervals $I_k$ (so that we have $m$ endpoints). Assume that $\frac 1{|I|}\int_I\log_-|f|\le\beta$ and $\frac 1{|I_k|}\left|\int_{I_k}f\right|<e^{-2\beta}$ for all $k$. Then $f$ has at least $\frac{m-1}2$ zero on $I$.

Proof. The inequality $\frac 1{|I_k|}\int_{I_k}\log_-|f|> 2\beta$ can hold for at most $\frac{m-1}2$ intervals $I_k$. For every remaining interval, we have $$ \frac 1{|I_k|}\int_{I_k}|f|\ge \exp\left[\frac 1{|I_k|}\int_{I_k}\log|f|\right] \\ \ge\exp\left[-\frac 1{|I_k|}\int_{I_k}\log_-|f|\right]\ge e^{-2\beta}>\frac 1{|I_k|}\left|\int_{I_k} f\right| $$ ensuring at least one crossing on $I_k$.

We have already estimated the average $\frac 1a\int_{[1-2a,1-a]}\log_-|P_n|$ by $C/a$ regardless of $n$. So now our task is just to make the integrals over $m-1$ its subintervals small. For some technical reasons to be explained below, it will be convenient to apply the above zero forcing lemma not to $P_n$ itself but to $f(x)=x^{L-1}P_n(x)$ with some integer $L>1$. Then $\frac 1{|I|}\int_I\log_-|f|\le \frac Ca+3La$, say, and if $x_j$ are the splitting points, we will just need to make the $m$ values $Q(x_j)$ of the polynomial $$ Q_n(x)=\frac 1L+\sum_{k=1}^n (-1)^k\frac{\varepsilon_k}{L+k} x^k $$ smaller than $e^{-2(C/a)-6La}\frac a{2(m-1)}$ (the antiderivative of $f$ is just $x^LQ(x)$). The most natural way to do it is to create an infinite series of the above kind converging to $0$ at each $x_j$. Notice that if this infinite series converges to $0$ at all, it does so at the geometric speed, more precisely, like $a^{-1}e^{-an}$ because $|x_j|^k\le e^{-ak}$ for all $j,k$ involved. So as soon as $n> 2Ca^{-2}+6L+a^{-1}\log[2(m-1)a^{-2}]$, we will be winning. In the end of the story $a$ will be of order $m^{-1}$ and $L$ be of order $m$, so we'll need to use $n$ of order $m^2$.

Greedy algorithm and trapping.

Let's first consider a simpler problem: creating a series $$ P(x)=1+\sum_{k=1}^\infty (-1)^k\varepsilon_k x^k $$ that converges to $0$ at just one point $x$. That can be achieved for $x$ close to $1$ by a simple greedy algorithm (take the next awailable term to go in the direction opposite to the current sum) but let us create a little theory about how and why this greedy algorithm works.

Let $A$ be the operator of multiplication by $1/x$ on the real line. Then our series is just $$ P(x)=1+\sum_{k=1}^\infty (-1)^k\varepsilon_k A^{-k}1\,. $$ For making it converge to $0$, it is enough to build a star-shaped with respect to the origin bounded trap $T$ such that we can force $P_n(x)\in A^{-n}T$ along some not too sparse sequence of $n$ (we'll use the arithmetic progression with even step $M$ starting at $0$). That, in turn, is feasible to do by a straitforward induction if $1\in T$ and we have the following decomposition property:

For every $v\in T$, and every even $n$, there exist $\varepsilon_{n+k}\in\{0,1\}$, $k=1,\dots,M$ such that $$ A^M v+\sum_{k=1}^M (-1)^k\varepsilon_{n+k} A^{M-k} 1\in T\,. $$ In this simplest case we can take $T=[-2,2]$, $M=2$ and, provided that $x$ is sufficiently close to $1$, make the following choice:

If $v\in [-1,1]$, put $\varepsilon_{n+1}=\varepsilon_{n+2}=0$;

If $v>1$, put $\varepsilon_{n+1}=1, \varepsilon_{n+2}=0$;

If $v<-1$, put $\varepsilon_{n+1}=0, \varepsilon_{n+2}=1$

Notice that for this choice not only shall we get back to $T$, but we will even get to $\frac 34T=[\frac 32,\frac 32]$. This contractive property is irrelevant now but will be important in the story about $Q$.

For the series $Q(x)$, we will demand that $Q_n(x)\in \frac 1{n+L}A^{-n}T$ instead. The decomposition property then changes to the following:

For every $v\in T$, and every even $n$, there exist $\varepsilon_{n+k}\in\{0,1\}$, $k=1,\dots,M$ such that $$ A^M v+\sum_{k=1}^M (-1)^k\varepsilon_{n+k} \frac{n+L}{n+k+L}A^{M-k} 1\in \frac{n+L}{n+M+L}T\,. $$

so now the contraction requirement is explicit. It gradually fades away but in the beginning, when $n=0$, it is crucial and dictates the presence and the choice of $L$. Since in our simple example we had the contraction factor of $\frac 34$, we must have $\frac{L}{L+M}=\frac L{L+2}\ge \frac 34$, i.e., $L\ge 6$ or something like that.

The case of $m$ points.

The reader shouldn't get suprised now that we introduce the diagonal linear operator $A=\operatorname{diag}[x_j^{-1}]$, consider the problem (we'll do $Q$ immediately) of finding the infinite series $$ Q=\frac 1L[1]+\sum_{k=1}^\infty (-1)^k\frac{\varepsilon_k}{L+k} A^{-k}[1] $$ converging to $[0]$ in $\mathbb R^m$ ($[\gamma]$ denotes the $m$-dimensional vector with all coordinates equal to $\gamma\in\mathbb R$) and reduce it to finding a star-shaped with respect to the origin bounded trap $T\in\mathbb R^m$ such that $[1]\in T$ and

For every $v\in T$, and every even $n$, there exist $\varepsilon_{n+k}\in\{0,1\}$, $k=1,\dots,M$ such that $$ A^M v+\sum_{k=1}^M (-1)^k\varepsilon_{n+k} \frac{n+L}{n+k+L}A^{M-k}[1]\in \frac{n+L}{n+M+L}T\,. $$

We will from now on use the notation $v_k=A^{-k}[1]$, so the last inclusion can be written as $$ A^M v+\sum_{k=1}^M (-1)^k\varepsilon_{n+k} \frac{n+L}{n+k+L}v_{k-M}\in \frac{n+L}{n+M+L}T\,. $$ The key to our proof is the following lemma by Newman (essentially the same lemma as in the Borwein-Erdelyi paper but in the discrete setting):

Newman's Decomposition Lemma

For every $\delta>0$, there is $\eta>0$ such that for every $x_j\in(0,1)$ ($j=1,\dots,m$) satisfying $\prod_j x_j>1-\eta$, there exist real coefficients $a_k$, $k\ge 0$ with $\sum_{k\ge 0}|a_k|<\delta$ and such that $$ v_{-1}-1=\sum_{k=0}^\infty a_k\xi^k v_k $$ where $\xi=e^{-\eta/m}$.

We will postpone the proof of the Newman decomposition lemma and its discussion to the end and now derive the desired inclusion from it assuming that $\delta>0$ is small enough (note that it will not depend on $m$ nevertheless, so $\eta>0$ we'll finally use will be just some absolute constant and we'll be able to choose $a=\frac{\eta}{2m}$ as promised).

First, observe that $\frac{n+L}{n+M+L}\ge \frac{L}{M+L}=\left(1 +\frac{M}{L}\right)^{-1}\ge e^{-\eta/m}=\xi$ if $L>\eta^{-1}M m$ (so, with fixed $M$, which will be just $2$ in the end, we can, indeed, choose $L$ to be a constant multiple of $m$ as promised). Thus, it will suffice to ensure that $$ A^M v+\sum_{k=1}^M (-1)^k\varepsilon_{n+k} \frac{n+L}{n+k+L}v_{k-M}\in \xi T\,. $$ We now fix the Newman decomposition and put $U_k=\sum_{\ell\ge k}|a_\ell|$, $k\ge 0$. Note that $\delta>U_0\ge U_1\ge\dots\ge 0$. We will be interested in the representations $$ v=\omega v_0+\sum_{k\ge 1}\lambda_k \xi^k v_k $$ with $\omega,\lambda_k\in\mathbb R$ and $|\lambda_k|\le\Lambda U_k$ where $\Lambda>0$ is some constant to be chosen later.

Using Newman's decomposition and taking a vector $v$ that allows the above representation, we can write $$ Av=\omega(v_{-1}-v_0)+(\omega+\xi\lambda_1)v_0+\xi\sum_{k\ge 1}\xi^k\lambda_{k+1}v_k \\ =(\omega+a_0\omega+\xi\lambda_1)v_0+\sum_{k\ge 1}\xi^k(\xi\lambda_{k+1}+\omega a_k)v_k \\ =\omega' v_0+\sum_{k\ge 1}\xi^k\lambda'_{k}v_k\,. $$ Note now that $$ |\lambda'_k|\le \xi \Lambda U_{k+1}+|\omega||a_k| \le\max(\xi\Lambda,|\omega|)(U_{k+1}+|a_k|)=\max(\xi\Lambda,|\omega|)U_k\,, $$ so if $|\omega|\le\xi \Lambda$, the resulting representation is not only again admissible, but improves by a factor of $\xi$. Of course, that improvement in $\lambda_k$'s comes at a cost of possibly increasing $|\omega|$, but that cost is not too high: we have $$ |\omega'-\omega|\le |a_0||\omega|+\xi\Lambda U_1\le \max(|\omega|,\xi\Lambda)(|a_0|+U_1) \\ =\max(|\omega|,\xi\Lambda)U_0\le\delta \max(|\omega|,\xi\Lambda)\,. $$ Let now $v(0)=v$ have an admissible representation with $|\omega|\le\Omega$ and let $$ v(m)=A^m v+\sum_{k=1}^m (-1)^k\varepsilon_{n+k} \frac{n+L}{n+k+L}v_{k-m}\,. $$ Then $v(m)=Av(m-1)+(-1)^k\varepsilon_k\frac{n+L}{n+m+L}v_0$.

Let $\omega_m$ be the coefficient at $v_0$ in the decomposition of $v(m)$. Then we have the recursion $$ \omega_m=\omega'_{m-1}+(-1)^k\epsilon_{n+k}\frac{n+L}{n+k+L} \\ =\omega_{m-1}+(-1)^k\varepsilon_{n+k}+E_m $$ where $$ |E_m|\le \delta \max(|\omega|,\xi\Lambda)+\frac{k}{n+k+L} \\ \le \delta \max(|\omega|,\xi\Lambda)+\frac M{M+L} \,. $$

Assume now that $|\omega_0|\le\Omega$, $\Omega\ge M(1+\delta\Lambda)$ and $2\Omega\le e^{-\eta}\Lambda$. Notice that the last two conditions can be easily satisfied by choosing any $\Omega>2M$, then any $\Lambda>6\Omega$ and, finally $\delta<\Lambda^{-1}$ and $\eta=\min(1,\eta(\delta)$ where $\eta(\delta)$ is given by the Newman decomposition lemma. In this case we can easily prove by induction that $|\omega_m|\le \Omega+2m\le 2\Omega$ for $m=0,\dots,M$ regardless of our choice of $\varepsilon_{n+k}$. Thus, we'll have all $v(m)$ having admissible representations and the estimate $$ |E_m|\le\delta\Lambda+\frac{M}{M+L}\,. $$ But then $$ \omega_M=\omega_0+\sum_{k=1}^M (-1)^k\varepsilon_{n+k}+E $$ where $|E|\le M\delta\Lambda+\frac{M^2}{M+L}<\frac 12$, provided that $\delta<\frac 1{4M\Lambda}$ and $L>4M^2$. It remains to note that if we take $M=2$ and $\Omega\ge 2$, then by choosing $\varepsilon_{n+k}$ appropriately (actually, just by pushing towards $0$ as hard as we can at each step), we can ensure that the expression on the right hand side is between $-\Omega+\frac 12$ and $\Omega-\frac 12$. If $\Omega-\frac 12<e^{-\eta}\Omega$ (another smallness condition for $\eta>0$), then we can use as our trap $T$ the set of all vectors $v$ having an admissible representation with $|\omega|\le\Omega$ and finish the proof modulo the Newman decomposition lemma.

Proof of the Newman decomposition lemma (compare to the argument in the Borwein-Erdelyi paper)

Define $$ B(z)=\prod_{j=1}^m\frac{1-\xi x_j z}{\xi x_j(\xi x_j-z) }, \quad Q_\ell(z)=1-\frac{\ell+1}{\ell}z^{-1}+\frac 1\ell z^{-\ell-1}\,. $$ with some positive integer $\ell$. Put $$ c_k=\oint_{|z|=1}B(z)Q_\ell(z)z^k\frac{dz}{2\pi i}\,. $$ Then we have $$ \sum_{k\ge 0}c_k\xi_k x_j^k= \oint_{|z|=1}B(z)Q_\ell(z)\frac 1{1-\xi x_j z}\frac{dz}{2\pi i}=-\frac{1}{\xi x_j} $$ by the residue theorem (move the contour out to $\infty$ and notice that the factor $1-\xi x_j z$ in the denominator cancels with the same factor in the numerator of $B(z)$, so there are no singularities outside the unit disk). Thus we can try to take $a_0=-\xi c_0-1$, $a_k=-\xi c_k$ for $k\ge 1$.

To show that $\sum_{k\ge 0}|a_k|$ is small, we can shift the contour to the circle $C$ with diameter $[-\frac 12,1]$, say. Note that on $C$, the ratio $\frac{|1-z|^2}{1-|z|}$ stays bounded and that $Q_\ell$ has a root of multiplicity $2$ at $1$, so $\frac{|Q_\ell(z)|}{1-|z|}$ is bounded on $C$ as well. Also, $|B(z)|\le |B(0)|=\prod_{j=1}^m\frac{1}{(\xi x_j)^2}\le e^{4\eta}$ and, thereby, we have $$ |c_k|\le C_k=e^{4\eta}\int_{C}|Q_\ell(z)||z|^k\frac{|dz|}{2\pi} $$ and $$ \sum_{k\ge 0}C_k=e^{4\eta}\int_{C}\frac{|Q_\ell(z)|}{1-|z|}\frac{|dz|}{2\pi}\le C(\ell)<+\infty\,. $$ Note also that the summable majorant $C_k$ does not depend on the choice of $x_j$, only on $\ell$ and $\eta$.

On the other hand, we have $$ \int_{|z|=1}|B(z)-1|^2 \frac{|dz|}{2\pi}=\int_{|z|=1}|B(z)|^2\frac{|dz|}{2\pi}-1\le e^{4\eta}-1\, $$ so as $\eta\to 0$, $B$ tends to $1$ at least in $L^2$ on the unit circle and, thereby, for every fixed $k\ge 0$, $$ c_k\to \oint_{|z|=1}Q_\ell(z)z^k\frac{dz}{2\pi i}=\begin{cases} -\frac{\ell+1}\ell, & k=0; \\ \frac 1\ell, &k=\ell; \\ 0 &\text{otherwise}; \end{cases} $$ and the dominated convergence theorem implies that as $\eta\to 0$, the sum $\sum_{k\ge 0}|a_k|\to\frac 2\ell$, which can be made as small as we want by choosing $\ell$ large enough.

That finishes my official answer. It is still a bit sketchy in places but I believe that it shouldn't be too hard to recover all the details. Nevertheless, feel free to ask as many questions as you need if something is still unclear. The Borwein-Erdelyi paper I referred to can be found here and the code for actually finding the polynomial with many roots (in Asymptote; we'll try to translate it into Mathematica later to work with higher precision required for $m>11$) is below. Thanks to everybody who managed to read up to this point for their attention and patience. I'll turn to something else now. :-)

int m=11;  int MM=40*m^2; int N=2^(floor(log(MM)/log(2))+5); int Q=MM;
real t=0.1;
real[] x;
int[] cf; cf[0]=1;
for(int k=0; k<m;++k) x[k]=exp(-(1.5+0.5cos(pik/(m-1)))*t/m);
pair zeta=(exp(-t/m),0);
real[] V; 
int l=16;
pair B(pair z)
{
pair s=(z-(l+1)/l+1/z^l/l); for(int i=0;i<m;++i) s=(1-zetax[i]z)/(zetax[i]-z)/zeta/x[i];
return s;
}
pair[] FF(pair[] u, int n)
{
pair[] v;
if(n==1) return copy(u);
pair[] ueven,uodd;
for(int k=0;k<n/2;++k){ueven[k]=u[2*k]; uodd[k]=u[2*k+1];}
int m=quotient(n,2);
pair[] veven=FF(ueven,m), vodd=FF(uodd,m);
for(int k=0;k<n;++k) v[k]=veven[k%m]+expi(2pik/n)*vodd[k%m];
return v;
}
pair[] UU;
for(int q=0;q<N;++q)
{
UU[q]=B(expi(2piq/N));
}
pair[] VV=-zeta/NFF(UU,N);
for(int k=0;k<N;++k) {VV[k]=zeta^k; V[k]=VV[k].x;}
V[0]-=1;
//pause();
real nm=0; for(int k=0;k<=Q;++k) nm+=abs(V[k]/zeta^k);
write(nm);
real a=x[1];
real s=0; for(int k=0;k<=Q;++k) s+=V[k]*a^k;
write(1/a-1,s);
int L=5*m;
real[] v; v[0]=1;
for(int s=1; s<=Q;++s) v[s]=0;
for(int mm=1;mm<MM;++mm)
{
real a=v[0]; for(int s=0;s<Q;++s) v[s]=v[s+1];
v[Q]=0;
v[0]+=a;
for(int i=0;i<=Q;++i) v[i]+=a*V[i];
real thresh=0;
if(v[0]>thresh && mm%2==1) {cf[mm]=-1;} 
else if(v[0]<-thresh && mm%2==0) {cf[mm]=1;}
else cf[mm]=0;
for(int s=0;s<=Q;++s) v[s]*=(mm+L)/(mm+L-1);
v[0]+=cf[mm];
if(mm%100==0) write(mm, v[0], (1-t/m)^mm);
}
import graph;
size(400,400,IgnoreAspect);
real f(real x) 
{
real s=0; for(int k=0;k<MM;++k) s+=cf[k]exp(-txk/m);//(k+L);
return 10.0^15s;
}
draw(graph(f,1,2,1000)^^(1,0)--(2,0)--(2,1));

The largest number of roots we can get with the standard float precision ($10^{-15}$) is 10 ($m=11$ anchor points). Beyond that the rounding errors dominate. If someone wants to try this code with higher precision, that would be interesting to see.

Answer 6

Using a different approach outlined below, I found the two dual polynomials of order $\nu=41$, \begin{align} P_7(x)&=x+x^{2}+x^{4}+x^{7}+x^{11}+x^{12}+x^{15}+x^{17}+x^{18}\\ &\quad{}+x^{20}+x^{24}+x^{29}+x^{30}+x^{31}+x^{35}+x^{38}+x^{40}+x^{41}\,, \tag{1a}\label{eq:1a}\\ \bar P_7(x)&=x+x^{2}+x^{4}+x^{7}+x^{11}+x^{12}+x^{13}+x^{18}+x^{22}\\ &\quad{}+x^{24}+x^{25}+x^{27}+x^{30}+x^{31}+x^{35}+x^{38}+x^{40}+x^{41}\,, \tag{1b}\label{eq:1b} \end{align} with $n=7$ distinct real roots, such that now $$\tag{2}\label{eq:2} \nu(7) \leq 41. $$ Note that $P_7(x) = x^{42} \bar P_7(1/x)$. The (restricted) search took only a few minutes on my iMac using Mathematica, code will be added below later.

I made the following assumptions:

1. I only considered $(b_k=0,1)$-polynomials
   $$\tag{3}\label{eq:3} P(x)=\sum_{k=0}^\nu b_k x^k $$ with $P(0)=P(-1)=0$, such that $b_0=0$ and $\sum_{k>0 \text{ even}} b_k=\sum_{k>0 \text{ odd}} b_k$. We have two trivial and $n-2$ nontrivial roots.
2. Define $b_{-k}=b_{\nu+1-k}$. From the OP's polynomials with $n<7$, I derived a pattern that with growing $n$ more and more of the first and last terms become symmetric, $$\tag{4}\label{eq:4} b_k=b_{-k} \,\, \forall \,\, k\leq k_n. $$ If this would hold for all $k$, it would give the palindromic polynomials used by @Peter Mueller.
3. The asymptotic set of nonvanishing $b_{k_m}=1$ for given $n$ is assumed to be defined by $$\tag{5}\label{eq:5} k_m=\frac{(m-1)\,m}{2}+1=\{1, 1, 2, 4, 7, 11, \ldots \}, \quad m=0,\ldots,n-2\,, $$ such that the distance between nonzero $b_k$ grows linearly, $k_{m+1}-k_m=m$. Therefore, $k_m-1$ are the triangular numbers.

For $n=7$ we get $k_{n-2}=11$, such that at $\nu=41$ $$\tag{6}\label{eq:6} P_7(x)=x+x^{2}+x^{4}+x^{7}+x^{11} +\left[\sum_{k=12}^{30} b_k x^k \right] +x^{31}+x^{35}+x^{38}+x^{40}+x^{41}, $$ which are only $75582$ instead of $2^{41}$ equations. From these, the two candidates in Eqs. (1) have $n=7$ distinct real roots. The polynomials with $\nu<41$ had a maximum of six roots, such that $\nu(7)=41$ under the assumptions 1-3. I conjecture that this is also correct without the assumptions.

For $n=6$, where $k_{n-2}=7$, the OP's polynomial does not have the assumed symmetry. However, for $\nu=28$ eight polynomials are found with this method and symmetry, from $3432$ candidates. One solution reads \begin{align}\tag{7}\label{eq:7} P_6(x)&=x + x^2 + x^4 + x^7 + (x^{13} + x^{15} + x^{16} + x^{18} + x^{20} + x^{21} )\\ &\quad {}+ x^{22} + x^{25} + x^{27} + x^{28}, \end{align} where the braces mark the "free" part.

The asymptotic series \begin{align} \tag{8a}\label{eq:8a} P_\infty(x)&=\sum_{m=1}^\infty x^{k_m}=\sum_{m=1}^\infty x^{\frac 1 2(m-1)m+1} \\ &=x + x^2 + x^4 + x^7 + x^{11} + x^{16} + x^{22} + x^{29} + \ldots \end{align} can be calculated for $\lvert x \rvert < 1$, with the result $$\tag{8b}\label{eq:8b} P_\infty(x)=\frac{1}{2} x^{7/8} \vartheta_2\left(0,\sqrt{x}\right)\,. $$ Here, $\vartheta$ denotes the Jacobi theta function, see the included plot.

Update 02.03.24

Well, there seems to be a grain of salt in this approach: Continuing to $n=8$ and $\nu=52$, with $184756$ equations, I cannot find a solution under the assumptions 1-3. It seems that $k_n$ grows too fast, leaving too few degrees of freedom ($DOF=20$) in $$ P_8(x)=x+x^{2}+x^{4}+x^{7}+x^{11}+x^{16} +\left[\sum_{k=17}^{36} b_k x^k \right] +x^{37}+x^{42}+x^{46}+x^{49}+x^{51}+x^{52}. $$