1

The wavefunction for an electron within a hydrogen atom in the $2s$ state has the following wavefunction:

$$\psi(r,\phi,\theta)=\psi(r)=\frac{1}{2\sqrt{\pi}}\left(2-\frac{r}{a_0}\right)\frac{e^{-r/2a_0}}{(2a_0)^{3/2}}$$

However, at $r=0$,

$$\psi^*\psi\left.\right|_{r=0}=\left(\frac{1}{2\sqrt{\pi}}\left(2-\frac{0}{a_0}\right)\frac{e^{-0/2a_0}}{(2a_0)^{3/2}}\right)^2=\frac{1}{\pi(2a_0)^{3}}$$

I don't understand how this should be possible. My answer doesn't match logic and it doesn't match graphs that I find online. (every graph I see goes to zero at $r=0$)

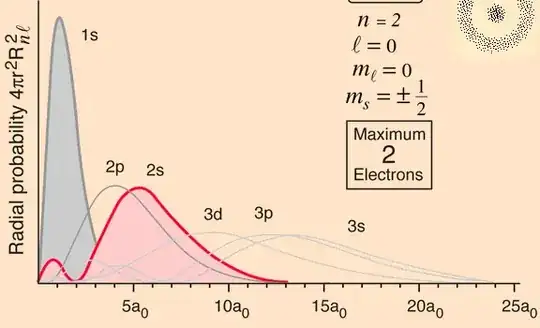
However, this does seem to really be the wavefunction for the 2s state.

Where have I gone wrong?

Arturo don Juan
  • 5,321
  • 1
    possible duplicate of Hydrogen radial wave function infinity at r=0 – m0nhawk Apr 07 '15 at 16:29
  • 1
    Why not? Especially the marked answer is a clear explanation that the probability isn't just a wavefunction at zero. You forget the volume, which a proportianal to $r^2$, so multiplying by zero is zero. And notice that the plot you added thave $4\pi r^2 R^2_{nl}$, and not just a wavefunction. – m0nhawk Apr 07 '15 at 16:32
  • Ah ok I see what you are saying. John's quick answer also helped. I thought it was different because I saw that that question was concerned with making sense out of a "diverging" wavefunction at $r=0$, whereas mine was about making sense of the fact that I got a nonzero value for the wavefunction at $r=0$ and the my assumption that the picture I put showed a contradiction. – Arturo don Juan Apr 07 '15 at 16:40

1 Answers1

8

The graph shows the probability of finding the electron between the distances $r$ and $r + dr$. This probability is given by:

$$ P = \psi^* \psi dV $$

where $dV$ is the volume element:

$$ dV = 4\pi r^2 dr $$

So we get the probability:

$$ P(r,r+dr) = \psi^* \psi 4\pi r^2 dr $$

and therefore when $r = 0$ the probability $P = 0$. It isn't that the wavefunction goes to zero at $r = 0$, but that the size of the volume element goes to zero.

John Rennie
  • 355,118
  • I'm still confused about this. I understand that the term describing the surface of a sphere goes to zero as $r$ does, but how do we reconcile that with the idea that the square of the wavefunction is the probability density? Because when you actually evaluate the wavefunction with $r = 0$, you get a positive value! It seems to me that the proper interpretation is that the radial distribution ceases to be a useful notion when $r = 0$, and that when talking about a single point in space, one must use only the square of the wavefunction. – Will Oct 28 '15 at 23:22
  • @Will: the radial distribution function shows the probability of finding the electron between the distances $r$ and $r+dr$. You say the RDF ceases to be useful when $r=0$, but this depends on how you define useful. The RDF is not the same as the electron density, but then it never claimed to be. It is a different function that is useful in different circumstances. – John Rennie Oct 29 '15 at 06:04
  • I see. Thanks! Then I have a question: I recently had an exam in chemistry which asked the question “What is the probability of finding an electron in the $2s$ orbital at the nucleus?” I had answered 0, but the answer was given later as “It depends on the atom.” So it seems that the right function to use in these circumstances is the square of the wavefunction, not the radial distribution—but I don't see exactly why. – Will Oct 29 '15 at 14:19
  • @Will: I assume the question was What is the probability of finding an electron in the 2s orbital inside* the nucleus?* otherwise it's a meaningless question. If so the answer is $\int_0^R P(r)dr = \int_0^R \psi^*(r) \psi(r) 4\pi r^2 dr$, where $R$ is the radius of the nucleus and $P(r)$ is the RDF. For the $2s$ orbital this will be non-zero. – John Rennie Oct 29 '15 at 14:54
  • That was the question verbatim, unfortunately. The “at” was a terrible choice of word; evidently they meant what you wrote. – Will Oct 29 '15 at 16:14