Why/How do the coefficients associated with atomic orbitals superposed to form hybrid orbital determine their spatial orientation?

Question

In my previous Phys.SE question, I asked for why $ \newcommand{\k}[2]{\langle #1|#2 \rangle} c_1,c_2,c_3,c_4$ in $$\psi_{sp^3}= c_1\psi_{2s}+ c_2\psi_{2p_{x}} + c_3\psi_{2p_y}+ c_4\psi_{2p_{z}}$$ would add to 1 when their squares are summed in order to ascertain whether hybridisation is a quantum superposition.

Now, I know that hybridised orbital is a quantum superposition which implies

$$c_1= \k{\psi_{2s}}{\psi_{sp^3}}, \\ c_2= \k{\psi_{2p_x}}{\psi_{sp^3}},\\ c_3= \k{\psi_{2p_y}}{\psi_{sp^3}},\\ c_4= \k{\psi_{2p_z}}{\psi_{sp^3}};$$ that is, each coefficient is the amplitude for the electron to go from $|\psi_{sp^3}\rangle$ to the respective atomic orbitals.

But, they are more than just amplitudes as said by Peter Atkins in his explanation:

The coefficients in the hybrid have been chosen to give the correct directional properties of the hybrid. The squares of the coefficients give the proportion of each atomic orbital in the hybrid.

Also, the diagram below also shows how for different values of coefficients, the spatial orientation is changed.

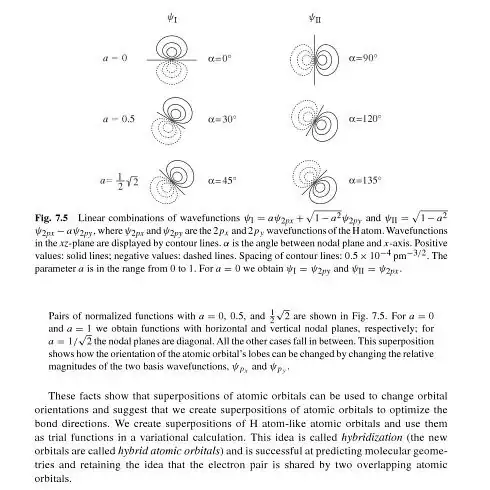
^{The snapshot above is taken from Principles of Physical Chemistry By Hans Kuhn, Horst-Dieter Försterling, David H. Waldeck}

I haven't found any deduction as to how the coefficients determine the spatial orientation. I thought they are just amplitudes to go from the hybridised state to the pure orbital state; how can they direct the spatial orientation? In fact, why would they have to direct the direction of the orbitals. They are probability amplitudes, aren't they? I just want to know how to deduce that these probability amplitudes are actually influencing the direction. How can it be deduced mathematically?

Answer 1

You start with:

$$ \psi_{sp^3}= c_1\psi_{2s}+ c_2\psi_{2p_{x}} + c_3\psi_{2p_y}+ c_4\psi_{2p_{z}} \tag{1} $$

Since $\psi_{sp^3}$ is normalised we know that:

$$ \langle \psi_{sp^3} | \psi_{sp^3} \rangle = 1 $$

and if we use equation (1) to substitute for $\psi_{sp^3}$ we get:

$$ \langle c_1\psi_{2s}+ c_2\psi_{2p_{x}} + c_3\psi_{2p_y}+ c_4\psi_{2p_{z}} | c_1\psi_{2s}+ c_2\psi_{2p_{x}} + c_3\psi_{2p_y}+ c_4\psi_{2p_{z}} \rangle = 1 $$

and expanding gives:

$$ \langle c_1\psi_{2s} | c_1\psi_{2s} \rangle + \langle c_1\psi_{2s} | c_2\psi_{2p_{x}} \rangle \, + ... + \, \langle c_4\psi_{2p_{z}} | c_3\psi_{2p_{y}} \rangle + \langle c_4\psi_{2p_{z}} | c_4\psi_{2p_{z}} \rangle = 1 $$

I've skipped the middle twelve terms because, well, life's too short and it should be obvious what they are. We can take the constants $c$ out of the brackets to get:

$$ c_1^2\langle \psi_{2s}|\psi_{2s} \rangle + c_1c_2\langle\psi_{2s}|\psi_{2p_{x}} \rangle \, + ... + \, c_4c_3\langle\psi_{2p_{z}} | \psi_{2p_{y}} \rangle + c_4^2\langle \psi_{2p_{z}} | \psi_{2p_{z}} \rangle = 1 $$

But the $2s$ and $2p$ orbitals are all orthonormal which means that $\langle\psi_i|\psi_j\rangle = \delta^i_j$, so our equation becomes:

$$ c_1^2 + c_2^2 + c_3^2 + c_4^2 = 1 $$

Which I think is what you were asking.

Response to comment:

The coefficients don't rotate anything, they are just numbers. What the diagram shows is that if you take two vectors:

then you can get a vector $\psi$ in any direction you want using:

$$ \psi = c_1\psi_1 + c_2 \psi_2 $$

with the normalisation condition $c_1^2 + c_2^2 = 1$. For example:

$$ \psi = \frac{1}{\sqrt{2}}\psi_1 + \frac{1}{\sqrt{2}}\psi_2 $$

is at 45º. More generally:

$$ \psi = \sin(\theta)\psi_1 + \cos(\theta)\psi_2 $$

gives you a vector at an angle $\theta$ to the horizontal, and it's automatically normalised since $\sin^2 + \cos^2 = 1$.

The diagram is just showing this applies to the $2p$ orbitals. Combining $2p_x$ and $2p_y$ in different proportions gives an orbital at different angles.

Answer 2

I honestly don't see why you're so mystified by this. Keep in mind that, in the end, you're adding up states which represent functions over real space, and these functions each have their own spatial dependence:

^{(Image source)}

The different colours represent different signs: blue is positive, red is negative. If you have a mixed wavefunction like, say, $$ \frac1{\sqrt{2}}\left(\psi_{2s}(\mathbf r)+\psi_{2p_z}(\mathbf r)\right) $$ then at $z>0$, where $\psi_{2p_z}(\mathbf r)>0$, the probability amplitude will increase, while at $z<0$ you have $\psi_{2p_z}(\mathbf r)<0$ so it detracts from $\psi_{2s}(\mathbf r)$ and the probability amplitude will decrease. The overall effect, then, is that the wavefunction's lump is pushed towards positive $z$. (Similarly, the orthogonal wavefunction $\tfrac1{\sqrt{2}}\left(\psi_{2s}(\mathbf r)-\psi_{2p_z}(\mathbf r)\right)$ is pushed towards negative $z$.

For the more general case of $sp^3$ mixing, with wavefunctions of the form $$\psi_{sp^3}(\mathbf r)= c_1\psi_{2s}(\mathbf r)+ c_2\psi_{2p_{x}}(\mathbf r) + c_3\psi_{2p_y}(\mathbf r)+ c_4\psi_{2p_{z}}(\mathbf r),$$ the $c_i$ act as probability amplitudes (in an abstract Hilbert-space view) but they also act as mixing coefficients between the different wavefunctions. Add some $\psi_{2p_{z}}$ and you push the orbital up; subtract some $\psi_{2p_{z}}$ and add some $\psi_{2p_{x}}$ and you push it down and forwards; and so on.