The angular momentum $L$ of an object is always defined with regards to the axis about which rotation takes place:
$$L=I\omega,$$
where $I$ is the inertial moment and $\omega$ the angular speed.
The inertial moment is defined and can be calculated as shown here. Where it's necessary to calculate $I$ with respect to another axis parallel to the first one, the Parallel Axis Theorem can be used.
Now with regards to angular momentum, analogous to linear momentum, it is a conserved quantity. In order to change linear momentum a force needs to be applied and in order to change angular momentum a torque (moment, couple [syn.]) needs to be applied.
How can we define angular momentum of a system about an axis that moves parallel to itself? For example Axis passing through CM that is perpendicular to plane of body.
I'm not sure I understand your question correctly. Angular momentum is always defined about an axis of rotation. If that axis itself moves the object will have both angular momentum $I\omega$ AND linear momentum $mv$ ($v$ is linear speed of that axis).
Look at the diagram below:
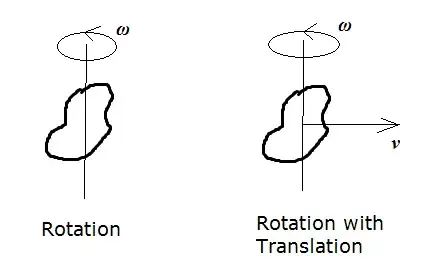
Left, the object rotates about an axis at constant $\omega$: pure rotation.
Right, the object rotates about an axis at constant $\omega$ and the axis moves parallel to itself at speed $v$: we have rotation and translation happening at once.
In both cases the angular momentum is exactly the same but the right object has linear momentum too.
The total kinetic energy of the object would be $K$:
$$K=\frac{I\omega^2}{2}+\frac{mv^2}{2}.$$
When we talk about conservation of momentum, then does it mean that angular momentum about a particular fixed axis remains same, or can we prove this for an axis that moves parallel too?
Yes. Even if that axis moves the angular momentum is conserved, until some torque acts on the object to alter its angular speed $\omega$.
An example is a cylinder rolling down a smooth slope: provided there's enough friction both angular and linear momentum will change because friction provides the couple $\sigma$ to alter $\omega$.
For example if a disk is falling freely. Can we say that Angular Momentum about an axis passing through CM and perpendicular to disk will remain same? How can we say this?
As long as no couple acts on the rotating disc, its angular speed and thus its angular momentum $L=I\omega$ will not change.
