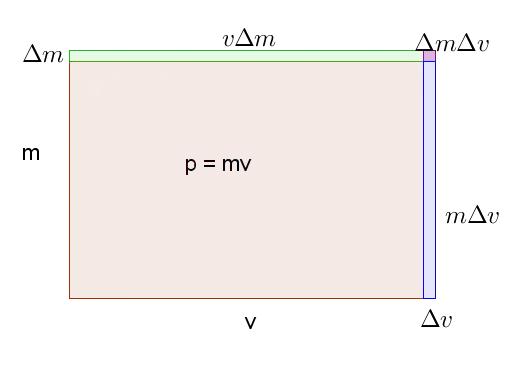
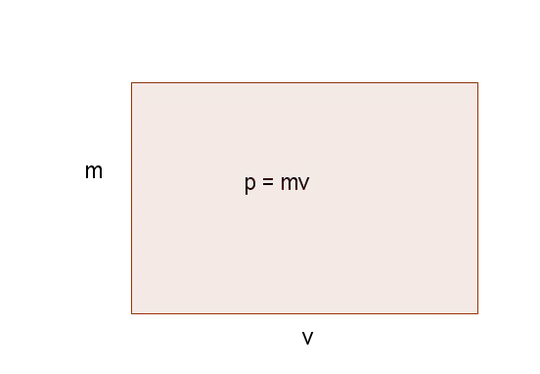That's how it's framed in my Physics school-book.
The question (or rather, the explanation) is that of the thrust of rockets and how the impulse is equal (with opposite signs) on the thrust-gases and the rocket itself.
$( m \frac { \Delta v}{ \Delta t} ) = -(v \frac {\Delta m}{ \Delta t} ) = F_i$
I suppose it's a problem with how I see the transfer of impulse and exactly which part of the equation relates to which part of the physical world (gases, rocket). So we can start from there.
Title equation:
$F = \frac{ \Delta (mv)}{ \Delta t} = \left ( m \frac { \Delta v}{ \Delta t} \right) + \left ( v \frac { \Delta m}{ \Delta t} \right)$
Grade: The equivalent of G-10 in the US.