Total number of distinct position and distinct momentum states in a finite volume

Question

I wrote an answer to this question on Distinct States, but I am not happy with the answer I gave to the short question at the end.

Hopefully this question is self contained, but please read the link above for full details, thanks.

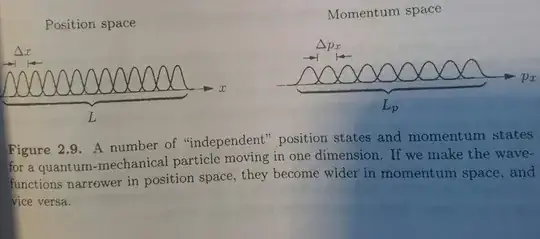
The problem is set in momentum space, and deals with the multiplicity of a monotonic ideal gas. From my textbook:

The number of independent wavefunctions is finite, if the total available position space and momentum space are limited.

L/$\Delta x$ is the number of distinct position states and L$_p$/$\Delta p$ is the number of distinct momentum states, (in a 1 dimensional system). The total number of distinct states is the product:

$\frac {L} {\Delta (x)}$$\frac {L_p} {\Delta (p )}$ =$\frac {LL_p} {h}$

Using the uncertainty principle.

My question is, what does this physically represent?

I am fairly familiar with Q.M., it is the momentum space concepts in thermodynamics that I am just starting to study.

My interpretation is that it simply represents the fact the particle could be at any distinct position within the space, and can have a distinct momentum at that position. So $X = 5$, $P = 7$, or any other combination of these distinct variables.

I self study, (with no one else to check with, so please excuse the confirm my idea aspect). If it's as simple as that explanation, I shall be excruciatingly embarrassed, but at least happy that I have it sorted out.

Answer 1

Mathematically, everything arises from the non-commutation of position and momentum. Practically, this means that there are no separate degrees of freedom for momentum and position in the wave function, as there is in the phase-space of classical statistical physics.

Ok, so here is a heuristic (many things will be very informal, the emphasis will be on intuition) justification to your equation:

Divide your system (particle in a periodic box of width L) to $N_x$ periodic boxes, so that new boxes have width $L/N_x$. There are infinite states in these small boxes as well, but their energy (and momentum) separation will be much larger due to their localization. The wave length of a wave fitting to a such of a box is now obviously $L/(N_x n)$, where n is an integer. The momentum is proportional to inverse wave length i.e. $N_x n /L$, and therefore, the separation of momentum is roughly $N_x/L$.

One notes immediately, that the more finely one defines the position grid, the further apart the expectation values of momenta are of these localized states. So, if we now say that we will impose a cutoff to momentum at $L_p$, roughly $Lp / (N_x / L)$ states will fit. So in total, one get's a total number of states, $N_x N_p = N_x \cdot Lp / (N_x / L) = L L_p$. And then there some factors of $\hbar$ and $2\pi$'s because I skipped the details.

More formally, one can take the original free wave solutions $\Psi_n({\bf r})$ of the box, and form new linear combinations $\Psi_{px} = \sum c_{pxn} \Psi_n({\bf r})$ which are localized to certain areas and have different momenta. They will never be exactly localized to a particular length $L/N_x$, but nevertheless, similar arguments can be followed through. The Heisenberg uncertainty principle does not care about the details, but it will always hold and give a limit to the maximum knowledge of momentum and position of the particle.

Answer 2

Let us for simplicity assume that the phase space is 2-dimensional. (There is a natural generalization to higher dimensions.) Semi-classically, the number of states $N(E)$ below energy-level $E$ is given by the area of phase space that is classically accessible, divided by Planck's constant $h$,

$$ N(E) ~\approx~ \iint_{H(x,p)\leq E} \frac{dx~dp}{h}. \tag{1}$$

(Here we ignore the Maslov index, also known as the metaplectic correction.) This shows that a compact phase space leads to a finite number of states. Formula (1) follows from the Bohr-Sommerfeld quantization formula, which can be derived from the WKB approximation, cf. e.g. this and this Phys.SE posts.