0

In SR, velocity addition allows one to get relative speeds for three objects, and they're all perfectly self-consistent.

The three objects are all moving collinearly, but differently. Any of the three relative speeds (between pairs of objects) can be derived by combining the other two speeds, using the SR velocity addition/subtraction formula.

So far so good. But then you convert those 3 relative speeds (between pairs of objects) into 3 relative time rates (between pairs of objects). And you find they're not consistent.

So suppose you have a lab with a clock in it, and you send two other clocks travelling off at different speeds, collinearly. Then bring them back to the lab (allowing for how they are moved), it's possible to account for the time dilation of each travelling clock in relation to the lab.

But it's not so easy, it seems, to account for the relative time rate between the two clocks that were sent off.

Or is there a way, if so, how is it done?

Thank you, David

user141183
  • 85
  • You may be confusing the differential of the rate at which time is observed to elapse due to a difference in velocity (time dilation) with the differential in accumulated clock time on different paths between two space-time events (accumulated interval as exemplified in the twin paradox). You need to understand these two issues separately, but they both work out with internal consistency. – dmckee --- ex-moderator kitten Feb 18 '18 at 21:06
  • Thanks. I do understand the difference. Assuming they all do a constant speed in a straight line, the factor difference is the same, whether time rate or accumulated clock time. So the time rates should be in a certain relationship. – user141183 Feb 18 '18 at 22:22
  • To phrase the question another way, is there a way to derive three time rates (not accumulated clock times), via sqrt (1 - [v/c]^2), from 3 velocities (that are found to be compatible via SR v addition formula), and find them to be compatible? (Presumably via factor differences)Thank you. – user141183 Feb 18 '18 at 22:41
  • As long as you "bring them back to the lab" you are building a twin-paradox type scenario, and the pure effect of time-dilation is complicated by the non-inertial nature of some of the paths. Showing that velocity composition and time dilation are compatible when dealing with multiple frames is a standard exercise and is only difficult in terms of bookkeeping and algebra, but to ask the question clearly you have to work with set of clocks prepared according to the Einstein synchronization procedure or some similar dodge to allow you to compare times at space-like separation. – dmckee --- ex-moderator kitten Feb 18 '18 at 23:04
  • Thank you - I don't need to bring them back to the lab. Shouldn't have put that, it complicated things unnecessarily. The question is simply about why the derived time rates are incompatible, as velocities generate time rates. A single relative v will lead to a single relative time rate. They should be compatible, surely, given that the velocities s are. Thanks, David – user141183 Feb 18 '18 at 23:16

1 Answers1

0

This is the famous twin paradox, but with triplets. Let's introduce two clocks A and B and send them away in the $x$-direction, but with different velocities, while we stay behind in the lab. At some point, the clocks must decelerate to get them back to the lab. In our frame, the worldlines of the clocks will look something like this:

enter image description here

As you say, it's possible to compute the elapsed time of the travelling clocks, and compare it to the lab clock. To be specific, the time measured by the clocks will be proportional to the length of their trajectories with respect to the Minkowski metric

$$ \mathrm{d}s^2 = -c^2\mathrm{d}t^2 + \mathrm{d}x^2. $$

The proper time is then given by $\mathrm{d}\tau^2 = -\mathrm{d}s^2/c^2$. Hence,

$$ \mathrm{d}\tau = \sqrt{1-\frac{1}{c^2}\frac{\mathrm{d}x^2}{\mathrm{d}t^2}}\mathrm{d}t = \sqrt{1-\frac{v^2}{c^2}}\mathrm{d}t. $$

Let's assume that both clocks depart at time $t_i$ and return at $t_f$ and that $|v_B|\geq |v_A|$ throughout. By dividing the elapsed times of clocks A and B, we obtain their "averaged relative rate":

$$ \frac{\Delta\tau_A}{\Delta\tau_B} = \frac{\int_{t_i}^{t_f}\sqrt{1-\frac{v_A^2}{c^2}}\mathrm{d}t}{\int_{t_i}^{t_f}\sqrt{1-\frac{v_B^2}{c^2}}\mathrm{d}t} \geq 1. $$

At any one moment, we also have the relative rate

$$ \frac{\mathrm{d}\tau_A}{\mathrm{d}\tau_B} = \frac{\sqrt{1-\frac{v_A^2}{c^2}}}{\sqrt{1-\frac{v_B^2}{c^2}}} \geq 1. $$

We thus see that the clock that travels at the lower velocity (A) runs faster, as expected. Of course, the lab clock runs even faster. You may actually be wondering about what happens when you move along with one of the travelling clocks. In that case, the answer should be the same, because we can simply compare our clocks at time $t_f$. However, your spacetime diagram looks like

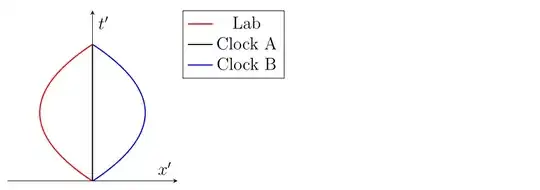
enter image description here

Based on this diagram, you might expect that the lab clock now runs slower than clock A. However, this is false, because lengths are measured differently in an accelerated reference frame. For the constant acceleration case depicted, you should use the Rindler metric. For the details of this computation, you should have a look at this answer.

Elbers
  • 26
  • My question is far simpler. It's just why doesn't this work - you get 3 relative velocities that all confirm each other. Then you convert them to relative time rates, via: sqrt [1 - (v/c)^2] . To see if they're compatible, you multiply and divide time rates, instead of adding and subtracting, as is done with velocities. If they're compatible, then any two should produce the third that way. Why doesn't it, that's the question. Thank you. – user141183 Feb 18 '18 at 22:16
  • Essentially, you're doing two subsequent Lorentz boosts. In that case, you have $t'' = \gamma_1(t' + \beta_1 x') = \gamma_1\gamma_2 t + \gamma_1\gamma_2\beta_1\beta_2 x$. This is equivalent to doing one Lorentz boost $t'' = \gamma(t+\beta x)$, provided that you use the velocity addition formula. So it's not valid to just multiply $\gamma$-factors, because you ignore the spatial part of the transformation. – Elbers Feb 18 '18 at 23:32
  • Thank you. Am I right in thinking that 'the spatial part of the transformation' is related to 'accumulated clock rate'? In other words, one needs to track what happens over a duration, rather than just compare γ-factors, which are more abstract? – user141183 Feb 18 '18 at 23:39
  • The transformation should have read $t''=\gamma_1\gamma_2(1+\beta_1\beta_2)t + \gamma_1\gamma_2(\beta_1+\beta_2)x$, sorry about that. It's not necessary to look at accumulated time. The point is that when you do a Lorentz transformation, you are mixing up your space and time axes, so you need to keep track of every coordinate that changes. To make it a bit more explicit, we can ask how much time elapses for someone who is stationary in $(t,x)$-coordinates for every unit of time in our $(t'',x'')$-coordinates. – Elbers Feb 19 '18 at 00:56
  • Since they are stationary, we set $\Delta x=0$, so $\Delta t'' = \gamma\Delta t + \gamma\beta\Delta x = \gamma\Delta t$. We can do the same calculation, by first going to $(t',x')$-coordinates. We find $\Delta t' = \gamma_2\Delta t + \gamma_2\beta_2\Delta x = \gamma_2\Delta t$. So far so good, but now $\Delta t'' = \gamma_1\Delta t' + \gamma_1\beta_1\Delta x'$. We cannot set $\Delta x'=0$, because they're not stationary in $(t',x')$-coordinates. This is the crux and why you'll ultimately find $\Delta t'' = \gamma_1\gamma_2(1+\beta_1\beta_2)\Delta t$. – Elbers Feb 19 '18 at 00:56