0

So in my classical mechanics book it states:

"For any sufficiently small displacement, any system of this kind behaves like a harmonic oscillator."

When discussing SHO. So I am curious what is considered to be "sufficiently small". Like with a spring I can extend it to a certain length and it will be so far from the equilibrium that deformation occurs and obviously at that point the spring will no longer behave as an oscillator, is this a good interpretation? I feel like it's not what is meant. I understand that Hooks law is derived from the maclourine series of potential energy and that because of that it has to be sufficiently close to equilibrium but what is considered "close"?

sammy gerbil
  • 27,277
chris360
  • 115
  • 1
    I believe it's the same as the pendulum. If you expand the sine function into a series, for small values of $\Theta$, you can stick on the first member of the series eg. $sin(\Theta)\approx \Theta$
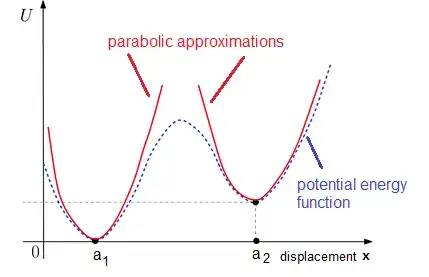
    I think that "close" is whenever the later terms of the series are negligible.
    http://people.math.sc.edu/girardi/m142/handouts/10sTaylorPolySeries.pdf     – Dominik Car Jul 24 '18 at 10:59
  • @Dominik Car, I believe you are right. Is there an example physical interpretation for the requirement of close? I don't know why I'm just stuck on the concept, mathematically it makes perfect sense but because with a spring using hooks law it doesn't "matter" in a theoretical sense what your initial displacement is wether it's 10mm or a meter you can still describe the force by -kx assuming no deformation occurs – chris360 Jul 24 '18 at 11:11
  • 1
    If the displacement is a length (rather than an angle as in the case of the pendulum), then there should be some characteristic length $l$ of the system comparing to which the displacement should be small. The Taylor series is then done not in $x$ but in the quotient $x/l$. – Photon Jul 24 '18 at 11:13
  • 1
    "Sufficiently small" depends on the characteristic scales of the physical system in question. Related: https://physics.stackexchange.com/q/408987/2451 , https://physics.stackexchange.com/q/159021/2451 and links therein. – Qmechanic Jul 24 '18 at 11:24
  • @Photon, ok so x is compared to l, which is the characteristic length of the system. My next question is do you have an example of a characteristic length? Like for a pendulum I assume the characteristic l is such that (x/l) > 0 or in my mind if x is zero than the potential energy comes to 2mgl and thus the pendulum will be in continuous circular motion. Is that an example of what is meant? – chris360 Jul 24 '18 at 11:25
  • 1
    For example, if you take the pendulum but parametrize it with the displacement $x$ from the equilibrium position rather than with the displacement angle $\theta$, then the length of the rope $l$ would be the characteristic length. – Photon Jul 24 '18 at 16:51
  • 1
    In simple words the equation of motion of a system is approximated. For example you might use x instead of sinx. These approximations make the equation linear. So the displacement should be small enough so that the approximated linear function is almost equal to the original function. So how small the displacement should be depends on the extent of approximation. – Saptarshi sarma Jul 24 '18 at 17:25

2 Answers2

5

You left out an important part of the quote :

Notice that this discussion applies to the motion of a particle near a stable equilibrium point of any potential energy function. For sufficiently small displacements, any system of this kind behaves like a harmonic oscillator.

Source: Classical Mechanics by Tom Kibble & Frank Berkshire, p 23

There is no universal definition of how small is "sufficiently small". It depends on the criterion you decide to apply. There is (in general) no definite point at which the behaviour of any stable oscillator suddenly becomes harmonic. It is only an approximation which gradually gets better the closer you get to the exact point of stable equilibrium. It is up to you to decide at what point deviations from ideal harmonic behaviour are insignificant.

What is being expressed here is the idea of a mathematical limit : that any potential energy function $U(x)$ can be approximated by a parabola $U(x)=\frac12 k(x-a)^2$ as accurately as you care to stipulate, provided that you limit yourself to a small enough range of $x$ around a point of stability $x=a$. Stability means that $U'(a)=0$ and $U''(a)>0$ - ie $x=a$ must be a local minimum of the function $U(x)$.

![![enter image description here

sammy gerbil
  • 27,277
  • It is impossible to define how small is "sufficiently small". - One could try something like: "sufficiently small enough that, to the precision one is working to, the system is indistinguishable from an ideal SHO" – Hal Hollis Jul 24 '18 at 15:15
2

Well, usually the idea of "sufficiently close" is relative to the dimensions of the other relevant quantities of the problem. For example, in the case of the spring you mentioned, one must take in consideration how its displacement compares to the length of the spring in its equilibrium state. When angles are involved, you can get a good estimate on the order of magnitude of the error by comparing the value obtained by second-order truncation of the Taylor Series of trigonometric functions with the "exact" value. This could be done with a simple calculator, but you can also look it up (the graphic on this Wikipedia, for example, contains information of this kind: https://en.wikipedia.org/wiki/Small-angle_approximation#Graphic).

That said, you're studying classical mechanics, so at this point I believe the most important thing is to get a physical understanding of oscillatory systems, and to this end the small-angle approximation is very good. Even when the error isn't negligible, the first non-zero term of a series expansion "dominates" the behavior of the function and, in particular, oscillatory systems that aren't really harmonic oscillators will behave like one in a small neighborhood of the equilibrium point.

Othin
  • 150