The entropic argument cited in a previous answer is probably the best account because it is the one that generalizes to $n$ dimensions.
For n dimensions, the corresponding quantity would be expressed in terms of the $n$-dimensional version of Newton's constant, $G_n$, as the $n-2$ Planck "area":
$$\frac{hG_n}{c^3} = \text{Planck } n-2 \text{ "area"}.$$
There are several observations that should be made about this that are almost universally neglected.
First, if the $4$-dimensional theory is an effective or dimensionally-reduced version of an $n$-dimensional theory, for $n > 4$, then it is actually the higher-dimensional Planck units which are fundamental, rather than the $4$ dimensional ones! The Planck length that comes out of the $n$-Planck area may be very different and on an entirely different scale than the Planck length that comes out of the $4$-Planck area. For instance, we may very well be on the threshold of new physics, if that quantity happens to be orders of magnitude larger than the Planck length!
Second, a simple dimensional analysis of the action integral for the Einstein-Hilbert action
$$
S = \int{\frac{R}{2κ}\sqrt{|g|}d^4x}, \\
[h] = [S] = \frac{[R] \left[\sqrt{|g|} d^4x\right]}{[κ]}, \\
[hκ] = [R] \left[\sqrt{|g|} d^4x\right] = \frac{1}{L^2} L^4 = L^2 = \left[\frac{hG}{c^3}\right], \\
[κ] = \left[\frac{G}{c^3}\right],
$$
shows (first of all) that the correct power of $c$ for the coupling coefficient $κ$ is $c^3$ not $c^4$ or $c^2$. All three appear in the literature, and $c^4$ appears in many on-line sites. These are wrong.
In 4 dimensions $κ = 8πG/c^3$, which generalizes in $n$ dimensions to
$$
κ_n = \frac{(n-1)(n-2)}{n-3} \frac{π^{½(n-1)}}{(½(n-1))!} \frac{G_n}{c^3} = 2π\frac{n-2}{n-3} \frac{π^{½(n-3)}}{(½(n-3))!} \frac{G_n}{c^3}.
$$
adopting the convention $(-½)! = \sqrt{π}$.
Second, and more importantly, it shows that the Planck area, when expressed as a function of $(h,κ,c)$
$$\frac{hG}{c^3} = ¼ħκ$$
or for the $n$-dimensional case in terms of $(h,κ_n,c)$
$$\frac{hG_n}{c^3} = \frac{n-3}{n-2} \frac{(½(n-3))!}{π^{½(n-3)}} ħκ_n$$
is independent of $c$!
Now, what does this mean?
Whatever theory is devised to integrate gravity with quantum theory will be based on an additional axiom that is independent of any notion of light speed and independent of the paradigm split between non-relativistic versus relativistic - and will apply equally well to Newtonian Gravity as it does to General Relativity.
Thus, such a theory can be found by combining Newtonian Gravity within the setting of Newton-Cartan chrono-geometries (or in the setting of a more comprehensive chrono-geometry that contains Newton-Cartan spacetimes) with quantum theory and proceeding from there.
The theory that combines general relativity with quantum theory must have this as its non-relativistic correspondence limit and must be derivable from it as a deformation in "theory space"; i.e. as a category-theoretic deformation of the category of quantized non-relativistic theories over either Newton-Cartan chrono-geometries or more comprehensive chrono-geometries that contains Newton-Cartan chrono-geometries into the category quantized general-relativistic theories over Lorentzian chrono-geometries.
The strongest hint as to what this theory should be, and what the additional axiom ought to be, lies with the fact that the Planck unit that comes directly out of combining the coupling $κ_n$ with $h$ is an $n-2$-area - the additional assumption should be something that involves $n-2$ areas and is something that may be posed generically for Newton-Cartan chrono-geometries, Lorentzian chrono-geometries (or even Riemannian, locally n-Euclidean, geometries or Carrollian chrono-geometries) and may, in fact, be an additional assumption that is generic to $n$-manifolds and to natural and gauge-natural bundles over $n$-manifolds. It is an additional axiom that may provide a quantum correction to, say, the classical diffeomorphism group over natural bundles and the classical gauge group over gauge-natural bundles.
My personal bias is that the additional axiom makes no adjustments to classical geometry at all, but only to the diffeomorphism and gauge groups: an axiom for "quantum" diffeomorphism/gauge transformations, i.e. a quantum correction to transformations that move space-time points.
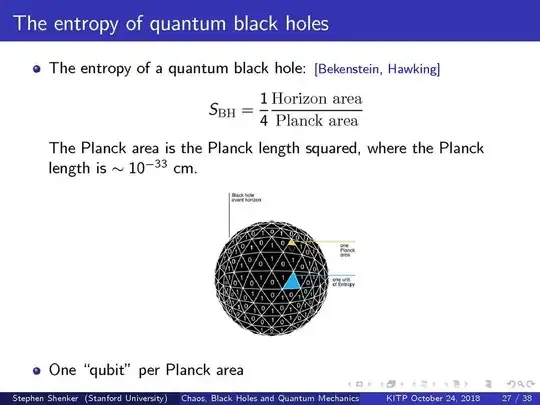 source
source