1

from engineering mechanics, by beer and johnston

For any system of n forces, we can simplify this system to a Force-Couple system. The simplification is intuitive- we don't need lot of forces just a Couple and Force. FIGURE 1

we can further simplify this force-couple system (which was already a simplification) in two ways.

  1. when $M$ is perpendicular to $R$. This is intuitive because we just need single force (no moment) (big savings!!) Figure 2.

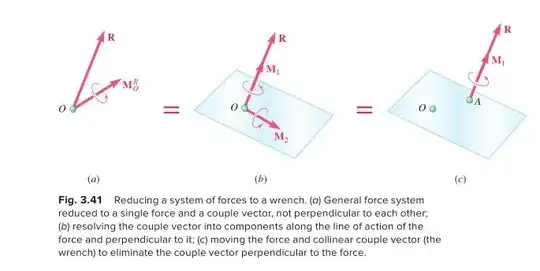
  2. In general case (converting it into a wrench). Figure 3

QUESTION: How is the second case -a simplification?

ark
  • 25
  • 2
    What simplification? What two systems? You need to provide more details to be understood. – Bob D Jan 23 '21 at 19:45
  • 2
    It still isn't clear. A wrench is a gadget for applying a torque to a nut. I don't understand what general force couple system you are talking about. I guess there are lots of ways to apply a force to a system. I don't see why a wrench is a simplification. Perhaps you mean that a wrench is a simple example of a gadget that applies force. But then I don't understand what you are asking. – mmesser314 Jan 23 '21 at 20:24
  • @BobD maybe now the question makes more sense. – ark Jan 23 '21 at 21:10
  • @mmesser314 it's called screw calculus , it was a system developed by somone named plucker which uses homogenous coordinates to simplfy calculations. – tryst with freedom Jan 23 '21 at 21:13
  • 1
    @Buraian Yeah man, screw calculus! (Ha Ha) – Bob D Jan 23 '21 at 21:18
  • @VIVEKSINGH Beer and Johnson. Wow. Had that book in my statics course 47 years ago! – Bob D Jan 23 '21 at 21:20
  • 1
    If I understood correctly, in the first case you simplify into one and shift it to a point such that the torque produced is same as original force , correct? If so, the second picture, you just shift it such that you kill off a component of the torque.. not sure why this would be useful though – tryst with freedom Jan 23 '21 at 21:24
  • Haha @BobD It's a serious field though, I saw it come when I was exploring robotics. Use a lot there it seems – tryst with freedom Jan 23 '21 at 21:25
  • yes, my man @Buraian gets it. – ark Jan 23 '21 at 21:31
  • @Buraian - Is this question clear enough that you could answer it? Not that you are going to. Just checking if it needs further clarification to avoid being closed. – mmesser314 Jan 24 '21 at 02:41
  • Does this answer your question? Understanding terms Twist and Wrench – John Alexiou Jan 24 '21 at 06:22

1 Answers1

2

A force and couple vectors need 3+3=6 components to fully describe them.

A wrench needs 3 components for the force, 3 components for the location (one of which is ignored, as force slide along their line) and 1 component for the scalar magnitude of the parallel torque along the line. The total is 7 components, 6 of which are actually used and are entirely equivalent to the first case.

But I think the book makes the statement by disregarding the notion that the location of the force line of action is needed, and thus left for 3+1 components which is indeed less than 6.

But the simplification of a wrench has to do with the fact that coordinate transformations are eliminated from the equations of motion, or the kinematics, as it is assumed that all wrenches and twists are expressed in the same coordinate system already.

So the static equilibrium of a body is expressed with wrenches as

$$ \sum_i \hat{f}_i = \hat{0} $$

where each wrench is $\hat{f}_i = \pmatrix{ \vec{F}_i \\ \vec{r}_i \times \vec{F}_i + \vec{\tau}_i } $

This is entirely analogous to the more perceived as complex vector form

$$ \begin{aligned} \sum_i \vec{F}_i &= \vec{0} \\ \sum_i \left( \vec{r}_i \times \vec{F}_i + \vec{\tau}_i \right) & = \vec{0} \end{aligned} $$

So even though there isn't an actual simplification, the notation is more compact, and when I write $\sum f_i$ I immediately understand it means summing all forces and moments about the origin without having write it out explicitly every time.

John Alexiou
  • 38,341