0

Can this constant be derived mathematically or is derived entirely from experimental data?

Mozibur Ullah
  • 12,994
Derek Seabrooke
  • 784

2 Answers2

5

It's derived experimentally, there is no known way to derive it mathematically.

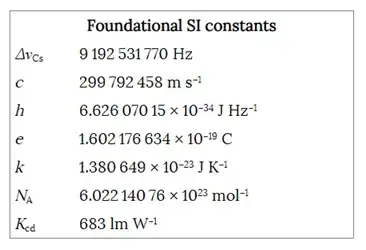
As many of the constants $c$, $h$ etc. are now defined to have definite values in the new SI system (see also question about $\mu_0$)

enter image description here

it really needs $\epsilon_0$ to be found experimentally, to determine the fine structure constant

as $$\alpha = \frac{e^2}{2 \epsilon_0 hc}$$

John Hunter
  • 13,700
  • Is e in your last calculation the elementary charge or Euler's constant? – Derek Seabrooke Aug 14 '21 at 15:21
  • @ Derek Seabrooke , the elementary charge, see also https://en.wikipedia.org/wiki/Fine-structure_constant – John Hunter Aug 14 '21 at 16:26
  • In this 2012 evaluation, $\alpha$ is computed from the measurement of the electron’s anomalous magnetic moment $\frac{g-2}{2}$. The largest uncertainties on $\alpha$ come from (1) the eighth-order Feynman diagrams, (2) the tenth-order Feynman diagrams, (3) high-energy hadronic and electroweak physics, and (4) the experimental uncertainty on $(g_e-2)$. – rob May 09 '22 at 02:36
4

In the standard model, the electromagnetic coupling is not a fundamental parameter. The electromagnetic interaction is just part of the more complicated electroweak interactions, the part that remains unaffected by the Higgs field, and the electromagnetic coupling has a Pythagorean dependence on the two fundamental electroweak couplings, see "e" in this diagram.

In practice, the electromagnetic coupling is inferred from various measurements, e.g. see section 10.2. of PDG's "Electroweak model" report.

There have been numerous attempts to find a mathematical formula for the fine-structure constant, but such a formula would need to arise in the context of a physical theory, to truly make sense. As I mentioned, there is a formula for it in the electroweak theory, "e = g sin theta_W", but that simply expresses its dependence on two other quantities which are free parameters of the standard model.

Some examples of what a theory-based formula might look like:

  1. If the electroweak interactions are united with the strong interaction in a grand unified theory, the fine-structure constant might be derived from a grand unified coupling equal to about 1/24 or 1/25, which could in turn come from a fundamental quantum geometry.
  2. Alternatively, the fine-structure constant can be expressed quite simply in terms of Feigenbaum's constant from dynamical systems theory (Manasson 2008, equation 11), so maybe it's a kind of infrared fixed point.
  3. Or maybe it's a parameter from the Deligne cohomology of the extra dimensions of string theory (see equation 6 forward, here).
Mitchell Porter
  • 13,121
  • Manasson's formula 2πδ² seems off by a few million sigma: 136.982510520... against 137.035999084 (21) per current CODATA.

    (Feigenbaum δ : https://oeis.org/A006890/constant, Sommerfeld 1/α : https://physics.nist.gov/cgi-bin/cuu/Value?alphinv)

     – Pallas Nov 04 '22 at 06:30
  • @Pallas Manasson's formula could just be an approximation. The exact prediction from a full-fledged theory realizing his idea could have some additional terms. In any case, no such theory exists for now. – Mitchell Porter Nov 05 '22 at 05:22