Suppose we have two objects $A$ and $B$ that are attached to each other. Suppose further there is a force $\vec{F}_{A}$ acting vertically on $A$ at $\vec{r}_{A}$ and there is a force $\vec{F}_{B}$ acting vertically acting on $B$ at $\vec{r}_{B}$. My main question is, can we combine these two forces into an equivalent resultant force $\vec{F}_{R}$ acting on the combined $A+B$ object at some position $\vec{r}_{R}$?
This post is relevant, but the replies there honestly don't resolve my specific quandary. I am dealing with a specific problem that I will outline in detail below.
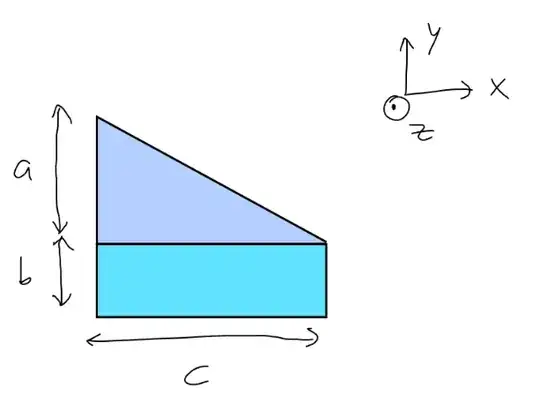
Suppose we have two objects $A$ and $B$ as shown below that are attached to one another.
We assume both objects have a uniform depth $d$ in the $z$-direction. Now suppose one sub-object experiences a force $\vec{F}_{A} = F_{A}\vec{e}_{y}$ acting at $\vec{r}_{A}$, and the other sub-object experiences a force $\vec{F}_{B} = F_{B}\vec{e}_{y}$ acting at $\vec{r}_{B}$, and these forces are possibly different (maybe for concreteness one part is magnetic and the other is not). This is shown below with point $O$ being the origin of coordinates.
Assuming $A$ and $B$ are attached to each other, I want to find the resultant force $\vec{F}_{R}$ acting on $\vec{r}_{R}$, as shown below.
To do this, I assume that $\vec{F}_{R}$ alone and the pair of forces $\vec{F}_{A}$, $\vec{F}_{B}$ both result in the same change in momentum and the same change in angular momentum:
\begin{align*} \vec{F}_{R} = \frac{d\vec{p}}{dt} = \vec{F}_{A} + \vec{F}_{B} \qquad\text{ and }\qquad \vec{\tau}_{R} = \frac{d\vec{L}}{dt} = \vec{\tau}_{A} + \vec{\tau}_{B}. \end{align*}
The first equation easily gives us
$$ \vec{F}_{R} = (F_{A} + F_{B})\vec{e}_{y}. $$
The second equation gives
\begin{align*} \vec{r}_{R}\times\vec{F}_{R} &= \vec{r}_{A}\times\vec{F}_{A} + \vec{r}_{B}\times\vec{F}_{B} \\ (r_{R, x}\cdot F_{R, y} - r_{R, y}\cdot 0) &= (r_{A, x}\cdot F_{A} - r_{A, y}\cdot 0) + (r_{B, x}\cdot F_{B} - r_{A, y}\cdot 0) \\ r_{R, x}F_{R, y} &= r_{A, x}F_{A} + r_{B, x}F_{B} \\ r_{R, x}(F_{A} + F_{B}) &= r_{A, x}F_{A} + r_{B, x}F_{B}. \end{align*}
Now $F_{A}$, $F_{B}$, $r_{A, x}$, and $r_{B, x}$ are all known, so then we can solve for $r_{R, x}$ and obtain
$$ r_{R, x} = \frac{r_{A, x}F_{A} + r_{B, x}F_{B}}{F_{A} + F_{B}}. $$
Now the last part is that I need to find $r_{R, y}$, but thinking about torque (or moment of forces) simply does not give us any info about $r_{R, y}$. Is the problem here underdetermined? If not, how can we deduce $r_{R, y}$?