Short exact sequences every mathematician should know

Question

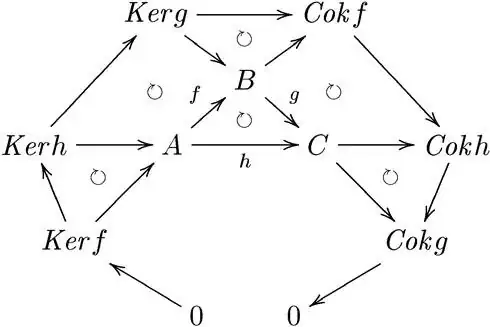
I'd like to have a big-list of "great" short exact sequences that capture some vital phenomena. I'm learning module theory, so I'd like to get a good stock of examples to think about. An elementary example I have in mind is the SES:

$$ 0 \rightarrow I \cap J \rightarrow I \oplus J \rightarrow I + J \rightarrow 0 $$

from which one can recover the rank-nullity theorem for vector spaces and the Chinese remainder theorem. I'm wondering what other 'bang-for-buck' short exact sequences exist which satisfy one of the criteria:

• They portray some deep relationship between the objects in the sequence that is non-obvious, or
• They describe an interesting relationship that is obvious, but is of important consequence.

Answer 1

There is one obvious sequence that underlies all vector analysis and a lot that builds up on it, no matter if its applied analysis, PDE, physics or the original foundations of algebraic topology. Yet it is rarely written out, as the people in the applied fields prefer to split it into its constituent statements and the people in pure mathematics are inclined to immediately write down some generalization instead. What I am talking about is of course the relationship between the classic differential operators on 3D vector fields:

$$0 \to \mathbb R\to C^\infty(\mathbb{R}^3;\mathbb{R}) \stackrel{\operatorname{grad}}{\to} C^\infty(\mathbb{R}^3;\mathbb{R}^3) \stackrel{\operatorname{curl}}{\to} C^\infty(\mathbb{R}^3;\mathbb{R}^3) \stackrel{\operatorname{div}}{\to} C^\infty(\mathbb{R}^3;\mathbb{R}) \to 0 $$

Answer 2

This might be very basic, but the short exact sequence $$ 0 \to \mathbb{Z} \to \mathbb{Q} \to \mathbb{Q}/\mathbb{Z} \to 0 $$ is both an injective resolution of $\mathbb{Z}$, and a flat resolution of $\mathbb{Q}/\mathbb{Z}$, making it a very useful exact sequence in many homological computations.

Answer 3

The exponential sheaf sequence: $$0\to 2\pi i\,\mathbb Z \to \mathcal O_M {\buildrel\exp\over\to}\mathcal O_M^*\to 0$$ where $\mathcal O_M$ is the sheaf of holomorphic functions on the complex manifold $M$, $\mathcal O_M^*$ is the sheaf of non-vanishing functions and $$\exp : \mathcal O_M \to \mathcal O_M^*$$ is induced by the usual exponential function.

Restricting to sections over any open set $U$ we get a long exact sequence including the map $$\cdots \to H^0(\mathcal O_U^*)\to H^1(2\pi i\,\mathbb Z|_U) \to \cdots.$$ The cohomology group $H^0(\mathcal O_U^*)$ is the set of non-vanishing holomorphic functions on $U$. Roughly speaking, the cohomology group $H^1(2\pi i\,\mathbb Z|_U)$ gives us ($2π i$ times) an integer for each closed loop in U. This map essentially tells us the winding number, around zero, of each non-vanishing holomorphic function, as we go around such a loop. I say all mathematicians should know this because the winding number is so fundamental. But this short exact sequence is also the starting point for a long path through all kinds of interesting places like the Riemann-Roch theorem.

Answer 4

I find it hard to believe that three days have gone by and no one has explicitly mentioned $$ 0 \to \Bbb Z \to \Bbb R \to \Bbb S^1 \to 0 $$

Answer 5

I think a short exact sequence that every teacher should know is

$$ 0 \to \mathbb R^d \to \mathrm{Isom}(\mathbb R^d) \to \mathrm{O}(\mathbb R^d) \to 0, $$

maybe for $d=2$ or $d=3$. Better still, forget about the origin and see $E=\mathbb R^d$ as an affine space.

It is a great visual helper, in the sense that many elementary properties take a concrete sense. It makes it clear that there is a copy of the group on the left hand side (the translations) in the isometry group, and that there is some other component described by the group on the right hand side, although how to perform the decomposition is not obivous (which is of course expected for working mathematicians, but a nice way to introduce it to others). Once we describe how the right hand side sits in the isometry group, it is very visual also that is not canonical, and that in fact there is a choice to be made to define such a copy, introducing what a section is and why it is interesting/important.

Answer 6

The short exact sequence

$$ 0 \to \mathrm{rad}({\mathfrak g}) \to {\mathfrak g} \to {\mathfrak g}/\mathrm{rad}({\mathfrak g}) \to 0$$

separates a Lie algebra ${\mathfrak g}$ into its solvable radical $\mathrm{rad}({\mathfrak g})$ and its semisimple projection ${\mathfrak g}/\mathrm{rad}({\mathfrak g})$, and is absolutely fundamental in the classification theory of Lie algebras, particularly in the characteristic zero, finite dimensional setting in which Levi's theorem is available to split the above sequence. Given the ubiquity of Lie algebras and Lie groups in mathematics, as well as the wider philosophy of separating algebraic objects into their "solvable" and "simple" components, I would submit that this sequence should be known to any mathematician.

In a somewhat similar spirit, the short exact sequence $$ 0 \to [G,G] \to G \to G/[G,G] \to 0$$ that separates a group $G$ into its commutator subgroup $[G,G]$ and its abelianisation $G/[G,G]$ is generally the first step towards understanding solvable groups $G$ (because if $G$ is solvable then $[G,G]$ is also solvable with the derived length decremented by one), while the analogous short exact sequence $$ 0 \to Z(G) \to G \to G/Z(G) \to 0$$ separating a group $G$ into its centre $Z(G)$ and the quotient $G/Z(G)$ is similarly often the first step in understanding nilpotent groups (because if $G$ is nilpotent then $G/Z(G)$ is nilpotent with the nilpotency degree decremented by one). There are of course analogues of these sequences for Lie algebras also.

In the category of topological (or algebraic) groups, one also has the short exact sequence $$ 0 \to G^0 \to G \to G/G^0 \to 0,$$ where $G^0$ is the identity component and $G/G^0$ is the totally disconnected projection. In principle, this separates the study of such groups into the connected and totally disconnected cases.

Answer 7

"Every mathematician should know" is too much to ask, but I do think the following is a great short exact sequence that captures a vital phenomenon: $$0 \to K(H) \to B(H) \to Q(H) \to 0.$$

$K(H)$ is the compact operators on a Hilbert space $H$, $B(H)$ is the bounded operators, and $Q(H)$ is the Calkin algebra. The "vital phenomenon" is that being invertible modulo the compacts, i.e., being Fredholm, is the same as being invertible in the Calkin algebra.

It won't help you learn module theory but IMHO it deserves to be on a big list ...

Answer 8

I strongly doubt there is any short exact sequence that every mathematician should know, but I certainly wish that those of them who know that for a (co)chain complex $(C,d)$ $$ 0\to\operatorname{Im}(d)\to\operatorname{Ker}(d)\to H(C,d)\to0 $$ is short exact, would also know that $$ 0\to H(C,d)\to\operatorname{Coker}(d)\to\operatorname{Im}(d)\to0 $$ is short exact too.

Answer 9

An example of a short exact sequence satisfying your first desiderata, but one which you probably won't fully understand till you are further along in homological algebra, is the Universal Coefficient Theorem. The homology version says: if $R$ is a PID, $A$ is an $R$-module, and $C$ is a flat chain complex over $R$, then there is a natural short exact sequence

$$ 0 \rightarrow H_n(C) \otimes_R A \rightarrow H_n(C\otimes_R A) \rightarrow Tor_1^R(H_{n-1}(C),A) \rightarrow 0 $$

Moreover, this sequence splits, and the splitting is natural in $A$ but not in $C$.

A related result is the Künneth Theorem: if $R$ is a PID and $X,Y$ topological spaces then there is a natural short exact sequence

$$ 0 \rightarrow \bigoplus_{i+j=k} H_i(X;R) \otimes_R H_j(Y;R) \rightarrow H_k(X\times Y; R) \rightarrow \bigoplus_{i+j=k-1} Tor_1^R(H_{i}(X;R),H_j(Y;R)) \rightarrow 0 $$

Furthermore, this sequence splits, but not canonically.

Answer 10

Within the category of Banach spaces and bounded linear maps,

$$0\to c_0 \to \ell_\infty \to \ell_\infty / c_0 \to 0$$

is a paradigm example of a short exact sequence that does not split, contrary to any short exact sequence

$$0\to c_0 \to X \to Z \to 0,$$

where $X$ (or $Z$) is separable. Here $c_0$ is the space of sequences convergent to 0 and $\ell_\infty$ is the space of bounded sequences, both endowed with the supremum norm.

The relevant results are Sobczyk's and Phillips-Sobczyk's theorems. See also the paper Sobczyk's Theorems from A to B by Félix Cabello Sánchez, Jesus M. F. Castillo, and David Yost.

Answer 11

How about the short exact sequence that expresses that every group can be expressed in terms of generators and relators? For any group $G$, there is a short exact sequence (in fact many) of the form $$0\to R\to F\to G\to 0,$$ with $F$ and $R$ being free groups. This expresses $G$ as a free group of generators modulo the relations encoded in $R$.

Of course there are analogous statements in other categories, such as those of modules.

Answer 12

For any abelian group $A$, there is a short-exact sequence $$0 \to T(A) \to A \to A/T(A) \to 0,$$ where $T(A)$ is the torsion subgroup of $A$, and $A/T(A)$ is torsion-free.

Answer 13

The kernel-cokernel exact sequence: in an abelian category, given $A \xrightarrow{f} B \xrightarrow{g} C$, the following sequence is exact

$$ 0 \to \ker f \to \ker gf \to \ker g \to \text{coker } f \to \text{coker }gf \to \text{coker} g \to 0$$

The maps are the obvious ones. The map $\ker g \to \text{coker } f$ is the one which factors through $B$.

I don't know if this fits, because it's not short and maybe it is too trivial, but I really think that every mathematician should know. For example, at a very low level, this tells the following basic facts

1. $gf$ is injective iff $f$ is injective and $\ker g \hookrightarrow{} \text{coker }f$
2. $gf$ is surjective iff $g$ is surjective and $\ker g \twoheadrightarrow \text{coker }f$
3. $gf$ is an isomorphism iff $f$ is injective, $g$ surjective and $\ker g \xrightarrow{\cong} \text{coker } f$
4. If $f$ and $g$ are injective/surjective, so is $gf$.

I think that more cools applications are covered in the following paper by Xiong, which I found just now.

A nice picture of this sequence from Nakaoka's website is the following

Answer 14

Given a finitely generated module $M$ over a commutative Noetherian ring $R$, there is a short exact sequence $$0\to M_1 \to R^n \to M\to 0$$ where you map $1$ in each $R$ to a generator of $M$ and $M_1$ (also finitely generated) is called a module of syzygy of $M$. Understanding this sequence (and it's repetitions) is a fundamental problem in commutative algebra and algebraic geometry and has generated countless beautiful results as well as been widely used a versatile tool on it's own. For instance, Hilbert's Syzygy Theorem says that if $R$ is a polynomial ring over a field, and you repeat this with $M_1$, etc, eventually you will get a free module.

Not sure there is one thing that "everyone should know", but for instance Stanley's proof of the Upper Bound Conjecture in combinatorics used a refinement of the Hilbert Theorem above.

Answer 15

I suppose many algebraic topologists would agree that the short exact sequence

$$0\longrightarrow \mathbb Z/p \longrightarrow \mathbb Z/p^2 \longrightarrow \mathbb Z/p\longrightarrow 0$$ giving rise to the Bockstein operator in (co)homology and the exact sequence $$ 0\longrightarrow C \stackrel{\cdot p}\longrightarrow C \longrightarrow C/pC\longrightarrow 0$$ giving rise to the Bockstein spectral sequence of the form $$H(C/pC) \Longrightarrow H(C)/pH(C)$$ may fit the bill. They are quite simple and lead to remarkably interesting mathematics.

Answer 16

The Tate extension. Let $k$ be a field, and let $V$ be the space $k((t))$ be the space of Laurent series with coefficients in $k$, considered as a topological vector space. If we write $\operatorname{GL}(V)$ for the group of (topological) automorphisms of $V$, then there is a canonical central extension

$$0\rightarrow k^{\times}\rightarrow\operatorname{GL}^{\flat}(V)\rightarrow\operatorname{GL}(V)\rightarrow0.$$

For a detailed construction, see http://www.its.caltech.edu/~justcamp/notes/sila.pdf. A brief summary: let $L$ be the space $k[[t]]\subset V$. Then for any element $g\in\operatorname{GL}(V)$, $gL$ and $L$ are commensurable (their intersection is of finite codimension in both) and so one can associate to them a one-dimensional vector space, the relative determinant. $\operatorname{GL}^{\flat}(V)$ can be defined as pairs of an element $g$ and a trivialization of the relative determinant.

Here the deep fact is the existence of the object $\operatorname{GL}^{\flat}(V)$. This leads to a host of other central extensions that are central (haha....) to the study of e.g. affine Lie algebras and friends (and thus to conformal field theory.) In a different direction, this short exact sequence is equivalent to the existence of the local residue symbol; in fact, I think Tate's name got attached to this because of his use of the local residue symbol to give a new proof of Riemann-Roch.

Answer 17

I saw $0\to \mathbb Z_p\to \mathbb Z_{p^2}\to \mathbb Z_p\to 0$ as an answer, with $p$ prime, but I will add with $p$ not prime and the particular choice $p=10$,

$$0\to \mathbb Z_{10}\to \mathbb Z_{100}\to \mathbb Z_{10}\to 0 $$ for the following reasons:

1. We all know that the group in the middle is given by $\mathbb Z_{10}\times \mathbb Z_{10}$ twisted by a 2-cocycle.

2. We know (if we don't, we should!) that the 2-cocyle is given by $f(n,m)=0$ if $n+m<10$ and $f(n,m)=1$ if $n+m\geq 10$, where $n,m\in\{0,\dots,9\}$.

3. Every child knows (or should know) (in particular, every mathematician -even a non algebraist one-) how to sum 2-digit numbers.

Since the question is what exact sequence we "should" know, I think 3. and 2. are two good reasons, even for the two different meanings of "should" know.

Answer 18

I guess the quintessential example, satisfying your second desiderata, is

$$ 0 \rightarrow A \stackrel{f}{\rightarrow} B \rightarrow B/f(A) \rightarrow 0. $$

For example, if $f = \mu_n: \mathbb{Z} \to \mathbb{Z}$ is multiplication by $n$, this means the following is exact

$$ 0 \rightarrow \mathbb{Z} \stackrel{\mu_n}{\rightarrow} \mathbb{Z} \rightarrow \mathbb{Z}/n\mathbb{Z} \rightarrow 0. $$

Another example of the same general result is that, if $C$ is finitely presented, then it fits in a short exact sequence

$$ 0 \rightarrow N \rightarrow P \rightarrow C \rightarrow 0 $$

where $N$ and $P$ are finitely generated, and $P$ is projective. Think of $P$ as the generators, and $N$ as the relations you quotient out by to get $C\cong P/N$.

Since you asked for a big list, I'll try to restrict myself to one example per answer.

Answer 19

Despite it being frequently used implicitly in papers (a classical example being Milnor's '56 paper about exotic spheres), I have never seen the following spelled out anywhere, so this might be a good place:

Let $\xi^n : E \to B$ be a real, smooth vector bundle over a manifold $B$. There is a short exact sequence of vector bundles over $E$,

$$0 \to \xi^*(\xi) \stackrel{i}{\to} \tau(E) \stackrel{d\xi}{\to} \xi^*(\tau(B)) \to 0,$$

where $i$ sends $(e_1, e_2) \in E \times_B E$ to the tangent vector starting at $e_1$ and pointing in the direction specified by $e_2$. Here, $\tau(M)$ denotes the tangent bundle of $M$. (As always, short exact sequences of vector bundles split.)

It follows from an easy dimension count. Alternatively, one can write out the gluing of the charts, giving something like

$$0 \to \text{colim } \mathbb R^n_\text{d} \times \mathbb R^n_\text{o} \times U_i \to \text{colim } \mathbb R^n_\text{d} \times \mathbb R^n_\text{o} \times TU_i \to \text{colim } \mathbb R^n_\text{o} \times TU_i \to 0,$$

where subscript $d$ and $o$ are just labels to distinguish between the coordinate which tracks the direction and that which tracks the origin, and $\{U_i\}$ is an atlas for $\xi$. This also gives a more rigorous description of $i$.

Answer 20

This one is just too much fun to leave out. Write the braid group on $n$ strands as $B_n$. By following the strands of a braid $\sigma\in B_n$ we construct a permutation of $n$ items, which we write as $\eta(\sigma)$. This $\eta$ is an epimorphism whose kernel is the pure braid group $P_n$. The pure braids are the braids whose strands end up where they started:

$$ P_n \to B_n \overset{\eta} \to S_n $$

Answer 21

Short exact sequences form a bridge of sorts between homological algebra and representation theory. For example, Maschke's theorem is the statement that, if $G$ is a finite group and $k$ is a field whose characteristic does not divide the order of $G$, then the $k$-representations of $G$ are completely reducible. This is equivalent to the statement that every short exact sequence of $k[G]$-modules

$$ 0 \rightarrow V_1 \rightarrow V_2 \rightarrow V_3 \rightarrow 0 $$

splits. This is, in turn, equivalent to the statement that $k[G]$ is a semisimple ring (there are many equivalent forms of what this means; my favorite is that every module is both injective and projective). You can then hit it with the Artin–Wedderburn theorem and write $k[G]$ as a product of matrix algebras. Strictly speaking, you don't need the language of short exact sequences, but many find it clarifying.

Answer 22

Another fundamental (half) short exact sequence is the Jacobi--Zariski sequence. For algebras over operads, for example, it takes the following form: for a triple $C\to B\to A$ of maps of $P$-algebras, there is a half exact sequence of functors $$0\longrightarrow \mathrm{Der}_B(A,-) \longrightarrow \mathrm{Der}_C(A,-) \longrightarrow\mathrm{Der}_C(B,-) $$ coming from the half exact sequences in Kahler differentials $$A\otimes_B\Omega_{B\mid C}^1\longrightarrow \Omega_{A\mid C}^1 \longrightarrow \Omega_{A\mid B}^1 \longrightarrow 0 $$ that is exact if $B\to A$ is a cofibration.

Answer 23

Decided to turn into an answer my comment to another answer here.

The Atiyah class $\alpha_E\in\operatorname{Ext}^1(E,\Omega^1\otimes E)$ of a holomorphic vector bundle $E$ is the class of the short exact sequence $$ 0\to\Omega^1\otimes E\to J^1(E)\to E\to0, $$ where $\Omega^1$ is the cotangent bundle (corresponding to the sheaf of holomorphic 1-forms) and $J^1(E)$ is the sheaf of first order jets of sections of $E$. A good reference is "Rozansky-Witten invariants via Atiyah classes" by Kapranov (Compositio Math. 115 (1999) 71-113). Kapranov notes that there is a dual way to represent this class, using another remarkable short exact sequence $$ 0\to E\to{\mathcal D}^{\leqslant1}\otimes_{\mathcal O}E\to T\otimes E\to0. $$ Here $T$ is the tangent bundle and ${\mathcal D}^{\leqslant1}$ is the sheaf of differential operators of order $\leqslant1$. (More precisely, this gives the class corresponding to $-\alpha_E$ in view of the canonical isomorphism $\operatorname{Hom}(-,\Omega^1\otimes-)\cong\operatorname{Hom}(T\otimes-,-)$.)

Answer 24

For a free product $A*B$ of groups $A$ and $B$, there is the exact sequence

$1 \to [A,B] \to A*B \to A \times B \to 1$

where $[A,B]$ is the subgroup generated by all elements $[a,b]=aba^{-1}b^{-1}$ and $A \times B$ is the direct product group. The first map is the inclusion and the second one is the intuitive one. This sequence is important for combinatorial and geometric group theory.

Answer 25

A starting point in anabelian geometry (a "thème central de la géométrie algébrique anabélienne", as Grothendieck writes in his Esquisse d'un Programme) can be considered to be the following:

Let $k$ be a field with separable closure $\overline{k}$ and $X$ a quasi-compact, quasi-separated $k$-scheme. If $\overline{x}$ is a geometric point of $X$ and the base change $X_{\overline{k}}$ is connected, then there is a short exact sequence of profinite topological groups:

$$ 1 \to \pi^{ét}_1(X_{\overline{k}}, \overline{x}) \to \pi^{ét}_1(X, \overline{x}) \to \pi^{ét}_1(Speck, \overline{x}) \simeq Gal(\overline{k}/k) \to 1$$

In fact, for $X=\mathbb{P}^1_{\mathbb{Q}}\backslash{\{0,1,\infty\}}$, J. S Milne on p. 30 of his LEC course notes, calls $\pi^{ét}_1(X, \overline{x})$ "arguably, the most interesting object in mathematics" due to the deep motivic ideas and profound connections that surround it, in relation with the already mysterious absolute Galois group of the rationals.

Answer 26

Let $M$ be a smooth manifold and $x:M\rightarrow \mathbb{R}$ a smooth function with $0$ as regular value, such that $X=\{x=0\}\subset M$ is a smooth submanifold. Then $$ 0\rightarrow x C^\infty(M)\hookrightarrow C^\infty(M) \xrightarrow{f\mapsto f\vert_X} C^\infty(X)\rightarrow 0 $$ is a short exact sequence and a right split corresponds to an extension map.

Where does this show up:

• For $M=\mathbb{R}$ this is the statement that the remainder in a Taylor series can be written as $R(x)=x^kr(x)$ for a smooth function $r(x)$.
• For $M=\mathbb{R}^d\times \bar {\mathbb{R}}^d$ (where $\bar{\mathbb{R}}^d$ is the radial compactification) and $x$ a boundary defining function of $\partial M=\mathbb{R}^d\times S^{d-1} = S^*\mathbb{R}^d$ (co-sphere bundle), this yields $$ 0\rightarrow \Psi_{\mathrm{cl}}^{m-1}(\mathbb{R}^d)\hookrightarrow \Psi_{\mathrm{cl}}^{m}(\mathbb{R}^d) \xrightarrow{\sigma_m} C^\infty(S^*\mathbb{R}^d)\rightarrow 0, $$ the shorth exact symbol sequence of pseudo-differential operators. Here $\Psi^m(\mathbb{R}^d)=\mathrm{Op}(x^{-m}C^\infty(M))$ with $\mathrm{Op}$ denoting the standard quantisation of symbols $a:\mathbb{R}^d_z\times \mathbb{R}^d_\xi\rightarrow \mathbb{C}$. One can take $x=\langle \xi \rangle^{-1}$ as bdf. of fibre-infinity. A right split is then a quantisation map. The symbol sequence (together with the multiplicativity of $\sigma_m$) allows to construct parametrices of elliptic operators and is thus the starting point of elliptic regularity theory.
• For $M=\bar {\mathbb{R}}^d\times \bar {\mathbb{R}}^d$, which is a manifold with corners, the constructions from the previous point yields Melrose's scattering (classical) scattering pseudo-differential operators.

Answer 27

Take a bundle $E \rightarrow M$ in $Diff$ and then apply the tangent functor. We get $TE\rightarrow TM$. The kernel of this is the vertical bundle, $VE$ and this all assembles into the short exact sequence:

$0 \rightarrow VE \rightarrow TE \rightarrow TM \rightarrow 0$

A splitting of this gives an Ehresmann connection, aka a horizontal bundle $HE$ such that $TE = VE \oplus HE$

The concept then descends to connections over vector bundles and principal bundles.

All this is in Michor, Kolar & Slovaks Natural Operations in Differential Geometry. It's possible to introduce curvature in this generality and which describes the local integrability of the horizontal bundle. In fact, they introduce it in greater generality over just a manifold, rather than a bundle and there they have cocurvature as well as curvature where the former describes the integrability of the vertical bundle. In the example above, the cocurvature vanishes as the vertical bundle is always integrable.

Answer 28

The following is both elementary and simple, and carries a useful content, so it may qualify at least as something most mathematicians may not regret to know. In the context of formal Laurent series,

$$0 \rightarrow \mathbb{C} \rightarrow \mathbb{C}((z)) \xrightarrow{D} \mathbb{C}((z)) \;\xrightarrow{ \mathrm{Res} }\; \mathbb{C} \rightarrow 0,$$

describes the main features of the operation of formal residue, taking a formal Laurent series $\sum_k c_kz^k$ to its coefficient $c_{-1}$. Among other results these allow to give an easy proof of the Lagrange inversion formula (check e.g. Genealogy of the Lagrange inversion theorem and its answers for other remarks).

Answer 29

This probably isn’t a short exact sequence that everyone needs to know, but it’s one of some significance for mathematical physics and differential geometry.

Let $G$ be a Lie group with Lie algebra $\mathfrak{g}$. Any smooth principal $G$-bundle $\pi : P \to B$ gives rise to a canonical short exact sequence $$ 0 \to \mathfrak{g} \times P \to TP \to \pi^\ast TB \to 0 $$ of $G$-equivariant vector bundles on the total space $P$, where $\mathfrak{g}$ carries the adjoint representation of $G$. Here, the map $\mathfrak{g} \times P \to TP$ is induced by the map that sends $X \in \mathfrak{g}$ to the corresponding fundamental vector field $X_P$ on $P$, while the map $TP \to \pi^\ast TB$ is given by $\pi_\ast$.

As Atiyah first observed, a principal connection can be identified with a splitting of this short exact sequence. Moreover, the gauge action of global gauge transformations on principal connections is compatible with this identification: if $f : P \to P$ is a gauge transformation and $\rho : \pi^\ast TB \to TP$ is a right splitting, then $f$ acts on $\rho$ to yield the right splitting $f_\ast \circ \rho$.

From the (somewhat idiosyncratic) perspective of noncommutative geometry, this short exact sequence can be viewed as relating the $G$-equivariant differential calculus on the total space $P$ to differential calculus on the base $B$ and the differential calculus along the orbits (modelled on that of the structure group $G$); a principal connection, then, tells you how to decompose the total differential calculus into a “direct sum” of basic and orbitwise calculi. Indeed, in noncommutative geometry itself, this short exact sequence—more precisely, a noncommutative analogue of its dual—actually becomes part of the definition of (algebraic) quantum principal bundle and principal connection.

Answer 30

A mathematician specialising in $C^*$-algebra theory should probably know the following short exact sequences. These play a central role in the $K$-theory of $C^*$-algebras.

• Let $A$ be a $C^*$-algebra with unitisation $A'= A \oplus \mathbb{C}$. There is an obvious split short exact sequence $$0 \to A \to A' \to \mathbb{C}\to 0$$ Hence, $K_i(A') \cong K_i(A)\oplus K_i(\mathbb{C})$.

• Let $\mathbb{A}$ be the Toeplitz algebra and $\mathbb{T}$ be the unit circle and $H^2$ be the Hardy space. Then we have a short exact sequence $$0 \to B_0(H^2) \to \mathbb{A}\to C(\mathbb{T})\to 0$$ This can be used to give a proof of the Bott-periodicity theorem.

• As mentioned in another answer, given a Hilbert space $H$, there is the short exact sequence $$0 \to B_0(H)\to B(H)\to B(H)/B_0(H)\to 0$$ which allows us to determine the $K$-theory of the Calkin algebra (via the six-terms exact sequence).

• If $\mathbb{S}=\{f\in C(\mathbb{T}): f(1)=0\}$, there is also a trivial split short exact sequence $$0 \to \mathbb{S}\to C(\mathbb{T})\to \mathbb{C}\to 0$$ which allows us to determine the $K$-theory of the $n$-dimensional torus $\mathbb{T}^n$, i.e. we can determine the $K$-theory of $C(\mathbb{T}^n)$.

Answer 31

I don't know whether this is a SES that every mathematician should know but it does satisfy the first sentence of the body of your question, since one could say it captures triangulability:

$$ 0 \to \text{ker}f \to \Theta_{3}^{H} \overset{f}{\to} \mathbb{Z}/2 \to 0 $$

where:

• the abelian group $\Theta_3^H$ is the cobordism group of oriented homology three spheres modulo binding an acylic PL/smooth 4-manifold.
• f is the Rokhlin homomorphism, which is 1/8th the signature of a compact, smooth spin(4) manifold that the integral homology sphere bounds.

Galewski, Stern and Matumoto showed in the 1980s that the non-splitting of this SES is equivalent to there being non-triangulable manifolds in every dimension 5 and above. Whereas, Manolescu recently showed that the SES does not in fact split.

Answer 32

A useful short exact sequence of sheaves in algebraic geometry is

$0 \rightarrow \mathcal{O}_C(K_C) \rightarrow \mathcal{O}_C(K_C + P) \rightarrow Q \rightarrow 0,$

where $Q$ is the quotient (a skyscraper sheaf with support in $P$).

Considering the long exact cohomology sequence associated to this short exact sequence leads to a standard proof of the Riemann-Roch formula for algebraic curves, since $L(C,K_C)$ is the space of global sections of the sheaf $\mathcal{O}_C(K_C)$ (see the textbook on Riemann surfaces by Otto Forster).

Answer 33

An explicit example that is my favourite is below which is in Humphreys book on Linear Algebraic Groups.

For a field $k$, let $T(n,k)$ denote the group of $n\times n$ non-singular upper triangular group and $D(n,k)$ the non-singular diagonal matrices and $U(n,k)$ upper triangular matrices with 1's in the diagonal.

$1\to U(n,k)\to T(n,k)\to D(n,k)\to 1$ is actually a split-sequence. This can be generalized to any connected solvable group leading to its structure theorem as semi-direct product of maximal unipotent normal subgroup and maximal torus subgroup.

Answer 34

Given an abelian category $\mathcal{A}$, and a diagram

$$\begin{matrix} W & \xrightarrow{f} & Y\\ \downarrow{g} & & \downarrow{h}\\ X & \xrightarrow{k} & Z\\ \end{matrix}$$

we can form an associated sequence

$$0 \to W \xrightarrow{(g,f)} X \oplus Y \xrightarrow{(k,-h)} Z \to 0$$

Then the diagram is:

0. Commutative iff the sequence is a complex,
1. Cartesian iff the sequence is a left exact complex,
2. co-Cartesian iff the sequence is right exact complex,
3. semi-Cartesian iff the sequence is a middle exact complex.

$\hphantom{0}$

Standard disclaimer: maybe not every mathematician, etc. etc., but it would behoove anyone working with commutative & homological algebra to be aware of this. It would be quite hoove indeed.

Answer 35

An example that might be useful in virtually all branches of mathematics: If $V$ and $W$ are vector spaces (over the same field $\mathbb{F}$) and if $U \subseteq V$ is a subspace, then the obvious exact sequence $$ 0 \longrightarrow U \longrightarrow V \longrightarrow V/U \longrightarrow 0 $$ turns into the non-trivial exact sequence $$ 0 \longrightarrow U \mathbin{\otimes} W \longrightarrow V \mathbin{\otimes} W \longrightarrow (V/U) \mathbin{\otimes} W \longrightarrow 0. \tag*{$(*)$} $$ (I rarely need exact sequences in my work, but simple manipulations like this make quotients and subspaces of tensor products much easier to deal with.)

Answer 36

The defining short exact sequence for Milnor's $K_2(R)$ ($R$ any ring) is

$$0\rightarrow K_2(R)\rightarrow St(R)\rightarrow E(R)\rightarrow 0$$

where $St(R)$ and $E(R)$ are the Steinberg and elementary groups. If we cheat a little on the definition of "short" this extends to

$$0\rightarrow K_2(R)\rightarrow St(R)\rightarrow GL(R)\rightarrow K_1(R)\rightarrow 0$$

Answer 37

From tensor of vector spaces, I find this exact sequence useful: $$0\rightarrow U\otimes V'+U'\otimes V\rightarrow U\otimes V \rightarrow (U/U')\otimes(V/V')\rightarrow 0. $$

For a commutative ring R and an element $x\in R$, denote $(0:x)=\{y\in R\colon yx=0\}$, I find this really good: $$0\rightarrow (0:x) \rightarrow R \rightarrow Rx\rightarrow 0 $$ It can be used to show that, in a local ring, we have: $$\mathrm{pd}_R(Rx)\leqslant 1 \iff Rx \text{ is projective } \iff x \text{ is not a zero-divisor} $$

Answer 38

The sequence $$0 \rightarrow \Psi^{\mu-1}(\Omega) \overset{\iota}{\rightarrow} \Psi^\mu(\Omega) \overset{\sigma_\mu}{\rightarrow}S_{h}^{\mu}(\Omega \times (\mathbb{R}^{n} \setminus 0)) \rightarrow 0$$ is exact, where $S_{h}^{\mu}(\Omega \times (\mathbb{R}^{n} \setminus 0))$ is the space of the functions $b:\Omega \times (\mathbb{R}^{n}\setminus 0) \rightarrow \mathbb{C}$ positively homogeneous of degree $\mu$, $\iota$ is the inclusion operator and $\sigma_\mu$ is the principal symbol of a pseudodifferential operador $B=op(b)$ with $b \in S^{\mu}(\Omega \times \mathbb{R}^{N})$.