How Does One Solve the Functional Root of $xe^x$

Question

Given the function $xe^x$ is there a way to solve the functional root, meaning solve for the function that satisfies the equation $f(f(x))=xe^x.$ I know that there may not be one unique solution that satisfies the functional root but in that case how do you find the family of solutions that does? (note, I'm looking for infinitely differentiable functions)

Answer 1

I'd like to give a practical computational technique for solving this and more general problems (but omitting proofs) as formal power series.

Suppose we have formal power series $f(x)=x+O(x^2)$. Use the notation $f^{\circ n}$ to mean $f$ iterated $n$ times, allowing $n$ to also take non-integral values.

Now consider what happens as we vary $n$ around $0$. We have a kind of flow on $\mathbb{R}$. Near $n=0$, $f^{\circ n}$ is approximately the identity function. So we expect $f^{\circ\varepsilon}(x) = x + \varepsilon g(x)+O(\varepsilon^2)$. This is a kind of infinitesimal flow, so we can think of $g(x) \frac \partial {\partial x}$ as a vector field on $\mathbb{R}$. Define the functional logarithm $\mathop{\mathrm{LOG}}(f)$ by $$f^{\circ\varepsilon}(x) = x+\varepsilon \mathop{\mathrm{LOG}}(f)(x) + O(\varepsilon^2)$$ so $g=\mathop{\mathrm{LOG}}(f)$.

The inverse of $\mathop{\mathrm{LOG}}$, call it $\mathop{\mathrm{EXP}}$, is the usual exponential of a vector field that turns an infinitesimal flow into a finite one, ie. $$\mathop{\mathrm{EXP}}(g)=\exp\left( g(x) \frac \partial {\partial x} \right)x$$

With these definitions we have $\mathop{\mathrm{EXP}}(\mathop{\mathrm{LOG}}(f))(x) = f(x)$ and more generally $\mathop{\mathrm{EXP}}(n\mathop{\mathrm{LOG}}(f))(x)=f^{\circ n}(x)$.

We can compute $\mathop{\mathrm{LOG}}(f)$ by analogy with the usual Taylor series for $\log(1+x)$.

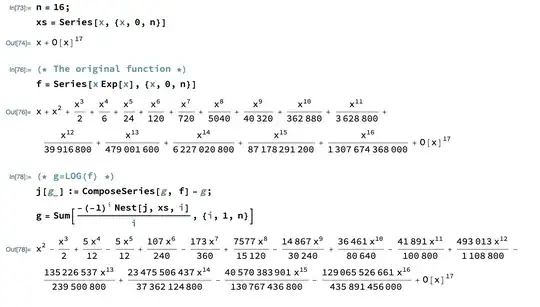
Just as we have (for suitable $x$) $$\log(x)=\sum_{i=1}^\infty-(-1)^i\frac{u^i}{i}$$ where $u=x-1$, it is possible to show (after some work) that $$\mathop{\mathrm{LOG}}(f)=-\sum_{i=1}^\infty(-1)^i\frac{j^{\circ i}(x)}{i}$$ where $j(g)=g\circ f-g$.

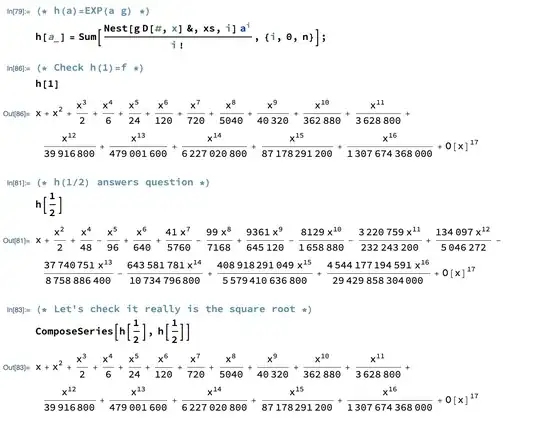
$\mathop{\mathrm{EXP}}$ is just the usual exponential of a vector field so it's given by $$\mathop{\mathrm{EXP}}(g)(x) = \sum_{i=0}^\infty \frac{1}{i!} \left(g(x) \frac \partial {\partial x} \right)^i x.$$

This now directly leads to a calculational method in Mathematica, say.

First the computation of the functional logarithm. Note how it's just one line in Mathematica.

Now exponentiate back up again, solving the OP's problem. Note again how the exponential is just one line.

As a bonus, our method gives us the series for the Lambert W function: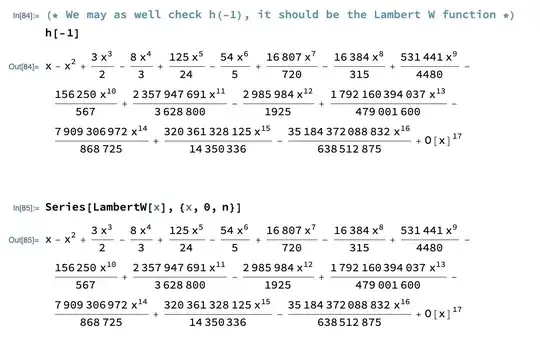

BTW Instead of computing the functional $\log$ via a series we could have computed functional square root directly using series. But I like this method as it solves a wide family of related problems.

PS I've given no thought to the convergence of any of these series.